Publications

Colonies of the bacteria Streptomyces coelicolor divide labour between cells that specialize on growth and sporulation and cells that specialize on antibiotic production. This division of labour arises due to costly chromosome deletions in the antibiotic overproducers. However, little is known about when and where these mutations occur or whether their frequency – which we liken to the caste ratio in social insects – is phenotypically plastic. To elucidate changes in the proportions of specialized cells (measured as the mutation frequency), we sampled S. coelicolor colonies grown under different conditions. Temporally, mutation frequency increased linearly with colony age and size. Spatially, mutations accumulated disproportionately in the colony center, despite greater growth and sporulation at the periphery. Exposing colonies to sub-inhibitory concentrations of some antibiotics, a competitive cue in Streptomyces, increased mutation frequencies. Finally, direct competition with other Streptomyces that naturally produce antibiotics increased mutation frequencies, while also increasing spore production. Our findings provide insights into the intrinsic and environmental factors driving division of labor in Streptomyces colonies by showing that mutation frequencies are dynamic and responsive to the competitive environment. These results show that chromosome deletions are phenotypically plastic and suggest that Streptomyces can flexibly adjust their caste ratio.

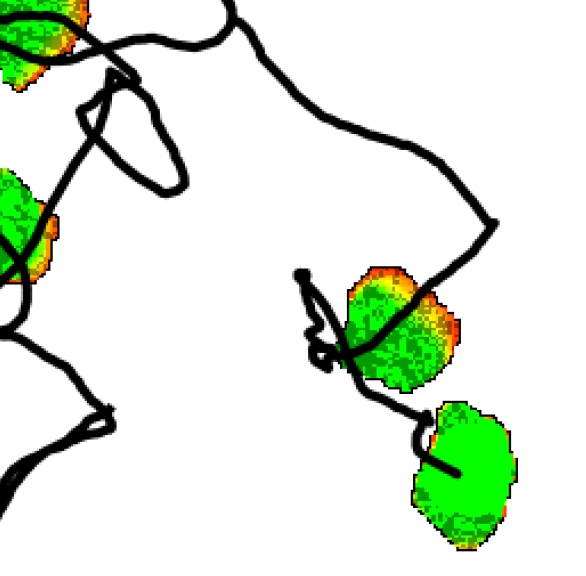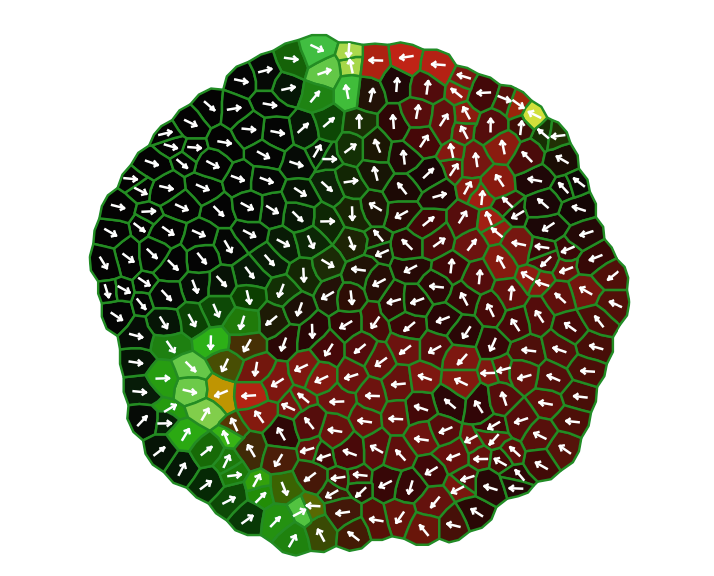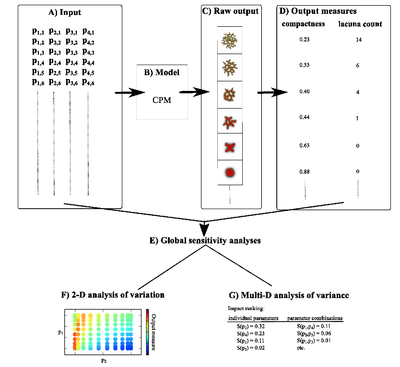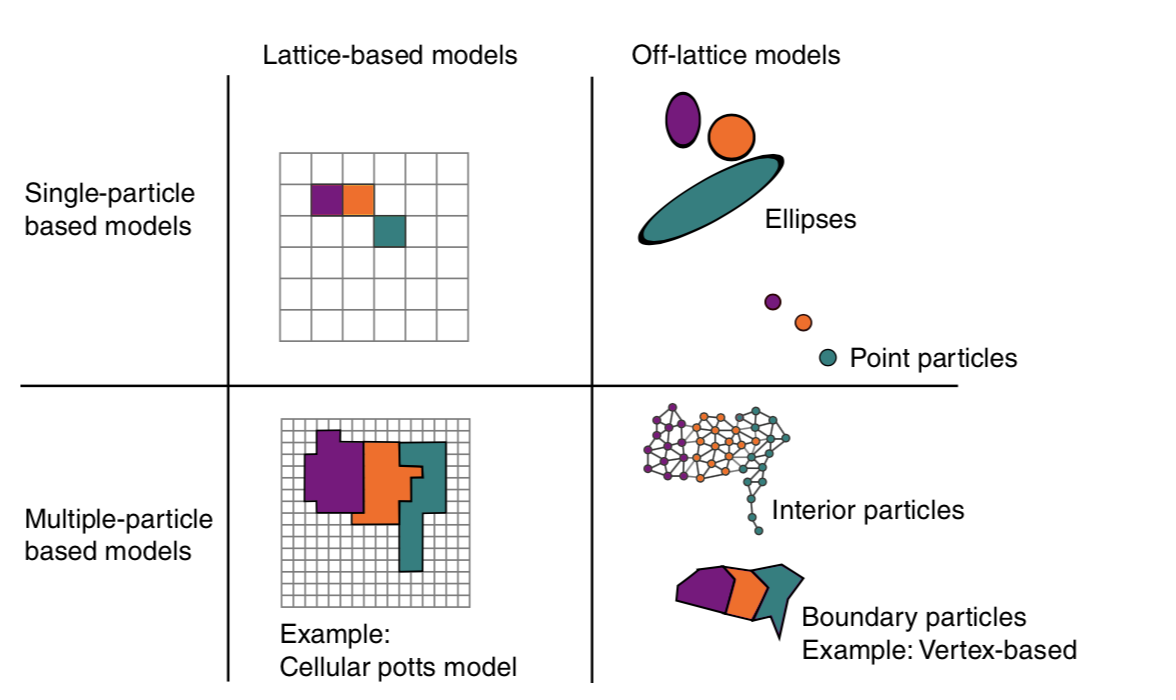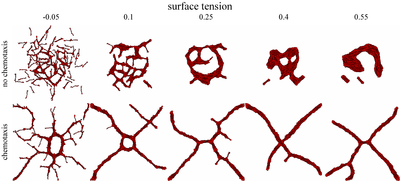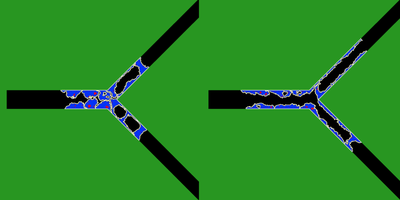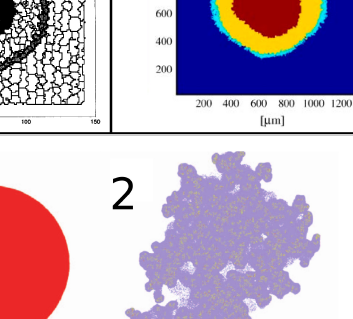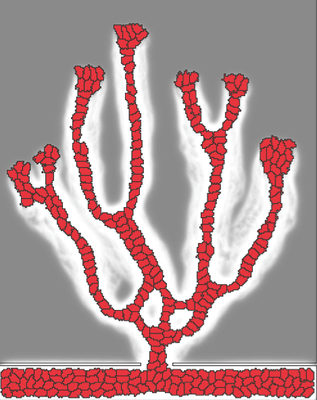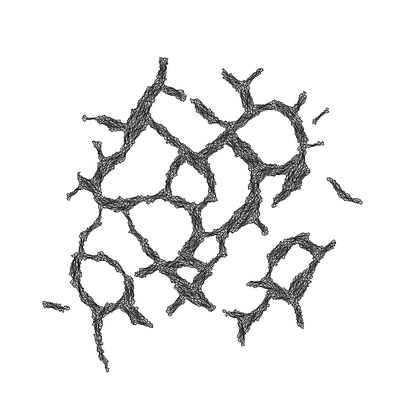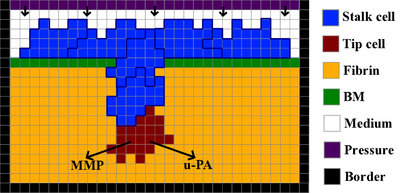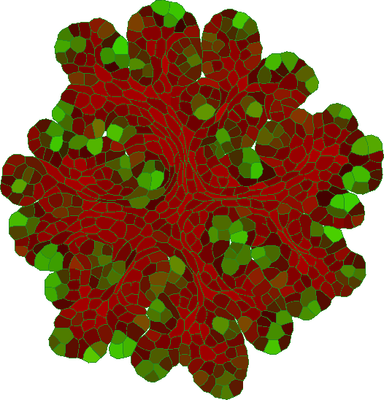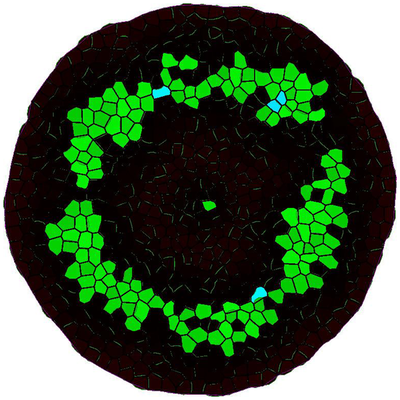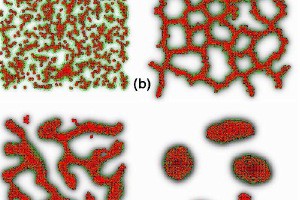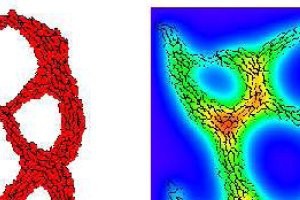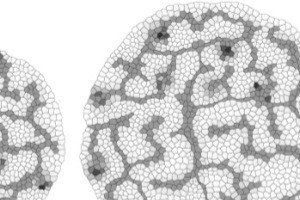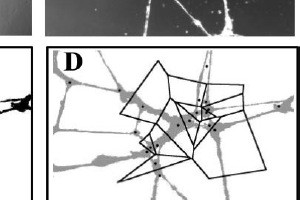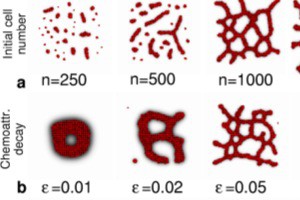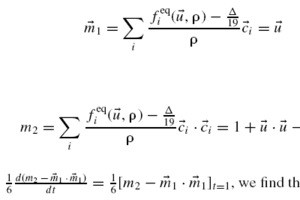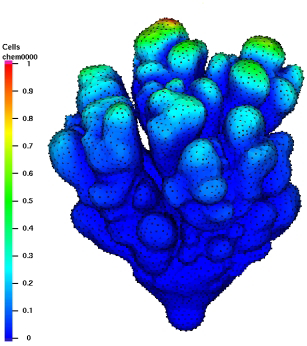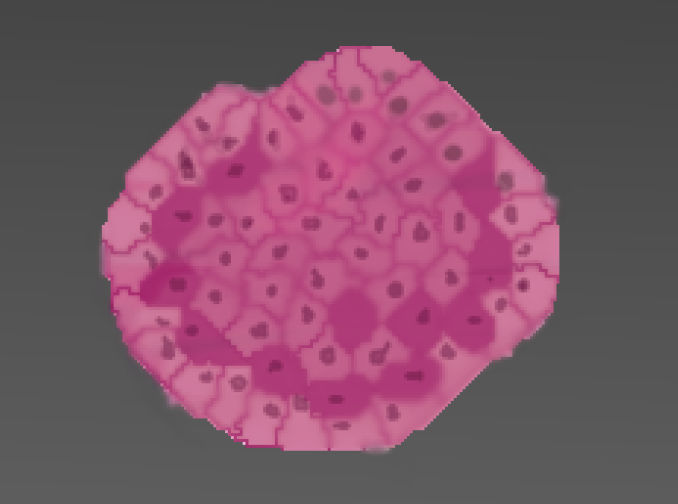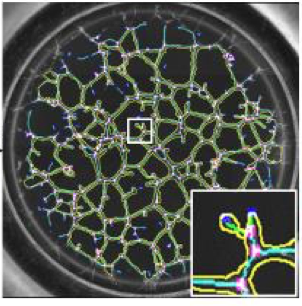
Abstract During angiogenesis, endothelial cells expand the vasculature by migrating from existing blood vessels, proliferating and collectively organizing into new capillaries. In vitro and in vivo experimentation is instrumental for identifying the molecular players and cell behaviour that regulate angiogenesis. Alongside experimental work, computational and mathematical models of endothelial cell network formation have helped to analyse if the current molecular and cellular understanding of endothelial cell behaviour is sufficient to explain the formation of endothelial cell networks. As input, the models take (a subset of) the current knowledge or hypotheses of single cell behaviour and capture it into a dynamical, mathematical description. As output, they predict the multicellular behaviour following from the actions of many individual cells, i.e., formation of a vascular-like network. Paradoxically, computational modelling based on different assumptions, i.e., completely different, sometimes non-intersecting sets of observed single cell behaviour, can reproduce the same angiogenesis-like multicellular behaviour, making it practically impossible to decide which, if any, of these models is correct. Here we present dynamical analyses of time-lapses of in vitro endothelial cell network formation experiments and compare these with dynamic analyses of three mathematical models: (1) the cell elongation model; (2) the contact-inhibited chemotaxis model; and (3) the mechanical cell-cell communication model. We extract a variety of dynamical characteristics of endothelial cell network formation using a custom time-lapse video analysis pipeline in ImageJ. We compare the dynamical network characteristics of the in vitro experiments to those of the cellular networks produced by the computational models. We test the response of the in silico dynamical cell network characteristics to changes in cell density and make related changes in the in vitro experiments. Of the three computational models that we have considered, the cell elongation model best captures the remodelling phase of in vitro endothelial cell network formation. Furthermore, in the in vitro model, the final size and number of lacunae in the network are independent of the initial cell density. This observation is also reproduced in the cell elongation model, but not in the other two models that we have considered. Altogether, we present an approach to model validation based on comparisons of time-resolved data and variations of model conditions.

Author Summary

Understanding how blood vessels grow and organise into well-structured networks is crucial for many clinical applications, from wound healing to cancer treatment. The growth of blood vessels, known as angiogenesis, involves endothelial cells migrating, proliferating, and forming new vascular structures. Angiogenesis is studied through biological experiments, but mathematical models are also used to test whether our understanding of cell behaviour is sufficient to explain cell network formation. Models assuming chemotaxis, elongated cell shape, contact inhibition or mechanical cell-cell communication often produce similar results despite the large differences in the underlying assumptions, making it difficult to determine which model is most accurate. In this study, we analysed time-lapse videos of endothelial cell network formation and compared these with three computational models: (1) the cell elongation model, (2) the contact-inhibited chemotaxis model, and (3) the mechanical cell-cell communication model. By examining the dynamics of network formation and testing how they change with cell density, we found that the cell elongation model best captures key aspects of the real-life cell network remodelling process. This approach highlights the importance of time-resolved data in evaluating computational models and provides a framework for refining our understanding of angiogenesis.

The ubiquitous Notch receptor signalling network is essential for tissue growth and maintenance. Operationally, receptor activity is regulated by two principal, counterposed mechanisms: intercellular Notch transactivation triggered by interactions between receptors and ligands expressed in neighbouring cells; intracellular cis inhibition mediated by ligands binding to receptors expressed in the same cell. Moreover, different Notch receptor/ligand combinations are known to elicit distinct molecular and cellular responses, and together, these phenomena determine the strength, the duration and the specificity of Notch receptor signalling. To date, it has been assumed that these processes involve discrete ligand homomers and not heteromeric complexes composed of more than one ligand species. In this study, we explore the molecular basis of the opposing actions of the Notch ligands, DLL4 and JAG1, which control angiogenic sprouting. Through a combination of experimental approaches and mathematical modelling, we provide evidence that two mechanisms could underpin this process: 1) DLL4 rather than JAG1 induces efficient Notch1 receptor transactivation; 2) JAG1 directly blocks DLL4-dependent cis-inhibition of Notch signalling through the formation of a JAG1/DLL4 complex. We propose a new model of Notch signalling that recapitulates the formation of tip and stalk cells, which is necessary for sprouting angiogenesis.

Many mammalian cells, including endothelial cells and fibroblasts, tend to align and elongate along the orientation of extracellular matrix (ECM) fibers in a gel when cultured in vitro. During cell elongation, clusters of focal adhesions (FAs) form near the poles of the elongating cells. FAs are mechanosensitive clusters of adhesions that grow under mechanical tension due to the cells' pulling on the ECM, and shrink when the tension is released. Here we use mathematical modeling to study the hypothesis that mechanical reciprocity between cells and the ECM suffices for directing cell shape changes and cell orientation. We show that FAs are preferentially stabilized along the orientation of ECM fibers, where the cells can generate more tension than perpendicular to the ECM fibers. We present a hybrid, computational model coupling three mathematical approaches. Firstly, the cellular Potts model describes an individual, contractile cell; secondly, molecular dynamics describe the ECM that is represented as a network of cross-linked deformable fibers; thirdly, a set of ODEs describes the dynamics of the cell's FAs, in terms of a balance between assembly and a mechanoresponsive disassembly. The resulting computational model shows that mechanical reciprocity suffices for stiffness-dependent cell spreading, local ECM remodeling, and ECM-alignment dependent cell elongation. These effects combined suffice to explain how cell morphology is determined by local ECM structure and mechanics.

Computational modelling has become increasingly important in advancing our understanding of biological systems, necessitating the development of new computational approaches and software. VirtualLeaf, in particular, is a modelling framework for plant tissues that accounts for the biophysical mechanics of plant cell interactions. The plant cell wall plays a pivotal role in plant development and survival, with younger cells generally having thinner, more flexible (primary) walls than older cells. Signalling processes in growth and pathogen infection also affect cell wall stability. This article presents an updated version of VirtualLeaf with improved cell wall mechanics and morphing behaviour. These are crucial for ultimately understanding plant tissue dynamics and essential signalling processes during growth, tissue formation and pathogen defence. The updated version of VirtualLeaf enables detailed modelling of variations in cell wall stability to the level of individual cell wall elements. These improvements lay the groundwork for using VirtualLeaf to address new research questions, including the structural implications of pathogen infection and growth.

Intestinal mucin acts as a barrier protecting the infant gut wall against diseases such as colitis and rotavirus. In vitro experiments have shown that the gut microbiota of breastfed infants consumes less mucin than the microbiota of non-breastfed infants, but the mechanisms are incompletely understood. The main difference between human milk and most infant formulas is the presence of human milk oligosaccharides (HMOs) in human milk. We hypothesize that HMOs protect mucin by stimulating non-mucin consuming bacteria. To understand the underlying mechanisms we developed a computational model that describes the metabolism and ecology of the infant gut microbiota. Model simulations suggest that extracellular digestion of the HMO 2'-fucosyllactose by the mucin-consumer Bifidobacterium bifidum may make this species vulnerable to competitors. The digestion products of HMOs become `public goods' that can be consumed by competing species such as Bacteroides vulgatus instead. Bifidobacterium longum, which does not consume mucin or produce public goods, can then become dominant, despite growing less efficiently on HMOs in monocultures than B. bifidum. In conclusion, our model simulations suggest that, through complex ecological interactions, HMOs may help lower mucin consumption by stimulating the non-mucin consumer B. longum at the expense of the mucin consumer B. bifidum.

Competing Interest Statement

This study was financially supported by FrieslandCampina. E.L., and J.M.W.G. are currently employed by FrieslandCampina.

The heartbeat is initiated by electrical pulses generated by a specialized patch of cells called the sinoatrial node (SAN), located on top of the right upper chamber, and then passed on to the atrium. Cardiac arrhythmias may arise if these electrical pulses fail to propagate toward the atrial cells. This computational modeling study asks how the morphology of the interface between sinoatrial (pacemaker) cells and atrial cells can influence the robustness of pulse propagation. Due to its strong negative potential, the atrium may suppress the pacemaker activity of the SAN if the electrical coupling between atrial cells is too strong. If the electrical coupling is too weak, however, the pacemaker cells cannot activate the atrial cells due to a source-sink mismatch. The SAN and atrium are connected through interdigitating structures, which are believed to contribute to the robustness of action potentials and potentially solve this trade-off. Here we investigate this interdigitation hypothesis using a hybrid model, integrating the cellular Potts model (CPM) for cellular morphology and partial-differential equations-based electrophysiological models for pulse propagation. Systematic examination of interdigitation patterns revealed that a symmetric geometry with medium-sized protrusions can prevent exit blocks. We conclude that interdigitation of SAN cells and atrial cells can promote robust propagation of action potentials toward the atrial tissue but only if the protrusions are of sufficient size and synchronicity of the action potential wave is maintained due to symmetry. This study not only highlights essential design principles for in vitro models of cardiac arrhythmias, but also provides insights into the occurrence of exit blocks in vivo.

A reduced capacity for butyrate production by the early infant gut microbiota is associated with negative health effects, such as inflammation and the development of allergies. Here we develop new hypotheses on the effect of the prebiotic galacto-oligosaccharides (GOS) or 2’-fucosyllactose (2’-FL) on butyrate production by the infant gut microbiota using a multiscale, spatiotemporal mathematical model of the infant gut. The model simulates a community of cross-feeding gut bacteria in metabolic detail. It represents the community as a grid of bacterial populations that exchange metabolites, using 20 different subspecies-specific metabolic networks taken from the AGORA database. The simulations predict that both GOS and 2’-FL promote the growth of Bifidobacterium, whereas butyrate producing bacteria are only consistently abundant in the presence of propane-1,2-diol, a product of 2’-FL metabolism. In absence of prebiotics or in presence of only GOS, however, Bacteroides vulgatus and Cutibacterium acnes outcompete butyrate producers by consuming intermediate metabolites.

Gastruloids have emerged as highly useful in vitro models of mammalian gastrulation. One of the most striking features of 3D gastruloids is their elongation, which mimics the extension of the embryonic anterior-posterior axis. Although axis extension is crucial for development, the underlying mechanism has not been fully elucidated in mammalian species. Gastruloids provide an opportunity to study this morphogenic process in vitro. Here, we measure and quantify the shapes of elongating gastruloids and show, by Cellular Potts model simulations based on a novel, optimized algorithm, that convergent extension, driven by a combination of active cell crawling and differential adhesion can explain the observed shapes. We reveal that differential adhesion alone is insufficient and also directly observe hallmarks of convergent extension by time-lapse imaging of gastruloids. Finally, we show that gastruloid elongation can be abrogated by inhibition of the Rho kinase pathway, which is involved in convergent extension in vivo. All in all, our study demonstrates, how gastruloids can be used to elucidate morphogenic processes in embryonic development.

Developmental time is a key life-history trait with large effects on Darwinian fitness. In many insects, developmental time is currently under strong selection to minimize ecological mismatches in seasonal timing induced by climate change. The genetic basis of responses to such selection, however, is poorly understood. To address this problem, we set up a long-term evolve-and-resequence experiment in the beetle Tribolium castaneum and selected replicate, outbred populations for fast or slow embryonic development. The response to this selection was substantial and embryonic developmental timing of the selection lines started to diverge during dorsal closure. Pooled whole-genome resequencing, gene expression analysis and an RNAi screen pinpoint a 222 bp deletion containing binding sites for Broad and Tramtrack upstream of the ecdysone degrading enzyme Cyp18a1 as a main target of selection. Using CRISPR/Cas9 to reconstruct this allele in the homogenous genetic background of a laboratory strain, we unravel how this single deletion advances the embryonic ecdysone peak inducing dorsal closure and show that this allele accelerates larval development but causes a trade-off with fecundity. Our study uncovers a life-history allele of large effect and reveals the evolvability of developmental time in a natural insect population.

The mechanical interaction between cells and the extracellular matrix (ECM) is fundamental to coordinate collective cell behavior in tissues. Relating individual cell-level mechanics to tissue-scale collective behavior is a challenge that cell-based models such as the cellular Potts model (CPM) are well-positioned to address. These models generally represent the ECM with mean-field approaches, which assume substrate homogeneity. This assumption breaks down with fibrous ECM, which has nontrivial structure and mechanics. Here, we extend the CPM with a bead-spring model of ECM fiber networks modeled using molecular dynamics. We model a contractile cell pulling with discrete focal adhesion-like sites on the fiber network and demonstrate agreement with experimental spatiotemporal fiber densification and displacement. We show that at high network cross-linking, contractile cell forces propagate over at least eight cell diameters, decaying with distance with power law exponent n= 0.35 – 0.65 typical of viscoelastic ECMs. Further, we use in silico atomic force microscopy to measure local cell-induced network stiffening consistent with experiments. Our model lays the foundation for investigating how local and long-ranged cell-ECM mechanobiology contributes to multicellular morphogenesis.

Significance

An open problem in mechanobiology is how cross talk between cells and extracellular matrix (ECM) translates to collective behavior. A major challenge is to understand how cells modify the ECM around them and how such modifications affect the cell itself and adjacent cells. Here, we developed an approach to simulate a cell interacting with a deformable ECM. We hybridized the widely used cellular Potts model with a bead-spring model of a fibrous, cross-linked ECM. Simulations of a contractile cell in an ECM fiber network reproduced and explained spatiotemporal experimental observations in cell culture, including long-range force propagation and observed scaling laws. The work enables future studies to unravel the coordinating role of the ECM in the development of tissues.

In vivo, cells navigate through complex environments filled with obstacles such as other cells and the extracel- lular matrix. Recently, the term ‘‘topotaxis’’ has been introduced for navigation along topographic cues such as obstacle density gradients. Experimental and mathematical efforts have analyzed topotaxis of single cells in pillared grids with pillar density gradients. A previous model based on active Brownian particles (ABPs) has shown that ABPs perform topotaxis, i.e., drift to- ward lower pillar densities, due to decreased effective persistence lengths at high pillar densities. The ABP model predicted topotactic drifts of up to 1% of the instantaneous speed, whereas drifts of up to 5% have been observed experimentally. We hypothesized that the discrepancy between the ABP and the experimental observations could be in 1) cell deformability and 2) more complex cell-pillar interactions. Here, we introduce a more detailed model of topotaxis based on the cellular Potts model (CPM). To model persistent cells we use the Act model, which mimics actin-polymerization-driven motility, and a hybrid CPM- ABP model. Model parameters were fitted to simulate the experimentally found motion of Dictyostelium discoideum on a flat surface. For starved D. discoideum, the topotactic drifts predicted by both CPM variants are closer to the experimental results than the previous ABP model due to a larger decrease in persistence length. Furthermore, the Act model outperformed the hybrid model in terms of topotactic efficiency, as it shows a larger reduction in effective persistence time in dense pillar grids. Also pillar adhesion can slow down cells and decrease topotaxis. For slow and less-persistent vegetative D. discoideum cells, both CPMs predicted a similar small topotactic drift. We conclude that deformable cell volume results in higher topotactic drift compared with ABPs, and that feedback of cell-pillar collisions on cell persistence increases drift only in highly persistent cells.

Significance

Knowing how the environment influences cell motility is useful in developing methods to interfere during disease or in tissue engineering. One factor is the presence of obstacles: in a process called topotaxis single cells move from a high to a low density of obstacles. Here, we show that a number of cellular properties, namely deformable volume, contact inhibition of locomotion, and adhesiveness to obstacles, influence the efficiency of topotaxis. Understanding the differences in these properties between cell types could point to cell sorting mechanisms for tissue engineering, or shed light on the migratory behavior of immune and cancer cells.

Precise organization of growing structures is a fundamental process in developmental biology. In plants, radial growth is mediated by the cambium, a stem cell niche continuously producing wood (xylem) and bast (phloem) in a strictly bidirectional manner. While this process contributes large parts to terrestrial biomass, cambium dynamics eludes direct experimental access due to obstacles in live cell imaging. Here, we present a cell-based computational model visualizing cambium activity and integrating the function of central cambium regulators. Performing iterative comparisons of plant and model anatomies, we conclude that the receptor-like kinase PXY and its ligand CLE41 are part of a minimal framework sufficient for instructing tissue organization. By integrating tissue-specific cell wall stiffness values, we moreover probe the influence of physical constraints on tissue geometry. Our model highlights the role of intercellular communication within the cambium and shows that a limited number of factors is sufficient to create radial growth by bidirectional tissue production.

Impact statement Radial plant growth produces large parts of terrestrial biomass and can be computationally simulated with the help of an instructive framework of intercellular communication loops.

Division of labor can evolve when social groups benefit from the functional specialisation of its members. Recently, a novel means of coordinating division of labor was found in the antibiotic-producing bacterium Streptomyces coelicolor, where functionally specialized cells are generated through large-scale genomic re-organisation. Here, we investigate how the evolution of a genome architecture enables such mutation-driven division of labor, using a multi-scale mathematical model of bacterial evolution. We let bacteria compete on the basis of their antibiotic production and growth rate in a spatially structured environment. Bacterial behavior is determined by the structure and composition of their genome, which encodes antibiotics, growth-promoting genes and fragile genomic loci that can induce chromosomal deletions. We find that a genomic organization evolves that partitions growth-promoting genes and antibiotic-coding genes to distinct parts of the genome, separated by fragile genomic loci. Mutations caused by these fragile sites mostly delete growth-promoting genes, generating antibiotic-producing mutants from non-producing (and weakly-producing) progenitors, in agreement with experimental observations. Mutants protect their colony from competitors but are themselves unable to replicate. We further show that this division of labor enhances the local competition between colonies by promoting antibiotic diversity. These results show that genomic organisation can co-evolve with genomic instabilities to enable reproductive division of labor.

Motivation of current work

Division of labor can evolve if trade-offs are present between different traits. To organize a division of labor, the genome architecture must evolve to enable differentiated cellular phenotypes. Cell differentiation may be coordinated through gene regulation, as occurs during embryonic development. Alternatively, when mutation rates are high, mutations themselves can guide cell and functional differentiation; however, how this evolves and is organized at the genome level remains unclear. Here, using a model of antibiotic-producing bacteria based on multicellular Streptomyces, we show that if antibiotic production trades-off with replication, genome architecture evolves to support a mutation-driven division of labor. These results are consistent with recent experimental observations and may underlie division of labour in many bacterial groups.

All tissue development and replenishment relies upon the breaking of symmetries leading to the morphological and operational differentiation of progenitor cells into more specialized cells. One of the main engines driving this process is the Notch signal transduction pathway, a ubiquitous signalling system found in the vast majority of metazoan cell types characterized to date. Broadly speaking, Notch receptor activity is governed by a balance between two processes: 1) intercellular Notch transactivation triggered via interactions between receptors and ligands expressed in neighbouring cells; 2) intracellular cis inhibition caused by ligands binding to receptors within the same cell. Additionally, recent reports have also unveiled evidence of cis activation. Whilst context-dependent Notch receptor clustering has been hypothesized, to date, Notch signalling has been assumed to involve an interplay between receptor and ligand monomers. In this study, we demonstrate biochemically, through a mutational analysis of DLL4, both in vitro and in tissue culture cells, that Notch ligands can efficiently self-associate. We found that the membrane proximal EGF-like repeat of DLL4 was necessary and sufficient to promote oligomerization/dimerization. Mechanistically, our experimental evidence supports the view that DLL4 ligand dimerization is specifically required for cis-inhibition of Notch receptor activity. To further substantiate these findings, we have adapted and extended existing ordinary differential equation-based models of Notch signalling to take account of the ligand dimerization-dependent cis-inhibition reported here. Our new model faithfully recapitulates our experimental data and improves predictions based upon published data. Collectively, our work favours a model in which net output following Notch receptor/ligand binding results from ligand monomer-driven Notch receptor transactivation (and cis activation) counterposed by ligand dimer-mediated cis-inhibition.

Author summary The growth and maintenance of tissues is a fundamental characteristic of metazoan life, controlled by a highly conserved core of cell signal transduction networks. One such pathway, the Notch signalling system, plays a unique role in these phenomena by orchestrating the generation of the phenotypic and genetic asymmetries which underlie tissue development and remodeling. At the molecular level, it achieves this via two specific types of receptor/ligand interaction: intercellular binding of receptors and ligands expressed in neighbouring cells, which triggers receptor activation (transactivation); intracellular receptor/ligand binding within the same cell which blocks receptor activation (cis inhibition). Together, these counterposed mechanisms determine the strength, the direction and the specificity of Notch signalling output. Whilst, the basic mechanisms of receptor transactivation have been delineated in some detail, the precise nature of cis inhibition has remained enigmatic. Through a combination of experimental approaches and computational modelling, in this study, we present a new model of Notch signalling in which ligand monomers promote Notch receptor transactivation, whereas cis inhibition is induced optimally via ligand dimers. This is the first model to include a concrete molecular distinction, in terms of ligand configuration, between the main branches of Notch signalling. Our model faithfully recapitulates both our presented experimental results as well as the recently published work of others, and provides a novel perspective for understanding Notch-regulated biological processes such as embryo development and angiogenesis.

The human intestinal microbiota starts to form immediately after birth, and is important for the health of the host. During the first days facultatively anaerobic bacterial species generally dominate, such as Enterobacteriaceae. These are succeeded by strictly anaerobic species, particularly Bifidobacterium species. An early transition to Bifidobacterium species is associated with health benefits: for example, Bifidobacterium species repress growth of pathogenic competitors and modulate the immune response. Succession to Bifidobacterium is thought to be due to consumption of intracolonic oxygen present in newborns by facultative anaerobes, including Enterobacteriaceae. To study if oxygen depletion suffices for the transition to Bifidobacterium species, here we introduce a multiscale mathematical model that considers metabolism, spatial bacterial population dynamics and cross-feeding. Using publicly available metabolic network data from the AGORA collection, the model simulates ab initio the competition of strictly and facultatively anaerobic species in a gut-like environment under the influence of lactose and oxygen. The model predicts that individual differences in intracolonic oxygen in newborn infants can explain the observed individual variation in succession to anaerobic species, in particular Bifidobacterium species. Bifidobacterium species become dominant in the model by using the bifid shunt, which allows Bifidobacterium to switch to sub-optimal yield metabolism with fast growth at high lactose concentrations as predicted here using flux-balance analysis. The computational model thus allows us to test the internal plausibility of hypotheses for bacterial colonization and succession in the infant colon.

Importance

The composition of the infant microbiota has a great impact on infant health, but its controlling factors are still incompletely understood. The frequently dominant anaerobic Bifidobacterium species benefit health, e.g., because they can keep harmful competitors under control and modulate the intestinal immune response. Controlling factors could include nutritional composition and intestinal mucus composition, as well as environmental factors, such as antibiotics. We introduce a modeling framework of a metabolically realistic intestinal microbial ecology in which hypothetical scenarios can be tested and compared. We present simulations that suggest that greater levels of intra-intestinal oxygenation more strongly delay the dominance of Bifidobacterium species, explaining the observed variety of microbial composition and demonstrating the use of the model for hypothesis generation. The framework allows us to test a variety of controlling factors, including intestinal mixing and transit time. Future versions will also include detailed modeling of oligosaccharide and mucin metabolism.

We analyze an 'up-the-gradient' model for the formation of transport channels of the phytohormone auxin, through auxin-mediated polarization of the PIN1 auxin transporter. We show that this model admits a family of travelling wave solutions that is parameterized by the height of the auxin-pulse. We uncover scaling relations for the speed and width of these waves and verify these rigorous results with numerical computations. In addition, we provide explicit expressions for the leading-order wave profiles, which allows the influence of the biological parameters in the problem to be readily identified. Our proofs are based on a generalization of the scaling principle developed by Friesecke and Pego to construct pulse solutions to the classic Fermi-Pasta-Ulam-Tsingou model, which describes a one-dimensional chain of coupled nonlinear springs.

Adhering cells exert traction forces on the underlying substrate. We numerically investigate the intimate relation between traction forces, the structure of the actin cytoskeleton, and the shape of cells adhering to adhesive micropatterned substrates. By combining the Cellular Potts Model with a model of cytoskeletal contractility, we reproduce prominent anisotropic features in previously published experimental data on fibroblasts, endothelial cells, and epithelial cells on adhesive micropatterned substrates. Our work highlights the role of cytoskeletal anisotropy in the generation of cellular traction forces, and provides a computational strategy for investigating stress fiber anisotropy in dynamical and multicellular settings.

In recent years, the mathematical and computational sciences have developed novel methodologies and insights that can aid in designing advanced bioreactors, microfluidic setups or organ-on-chip devices, in optimizing culture conditions, or predicting long-term behavior of engineered tissues in vivo. In this review, we introduce the concept of computational models and how they can be integrated in an interdisciplinary workflow for Tissue Engineering and Regenerative Medicine (TERM). We specifically aim this review of general concepts and examples at experimental scientists with little or no computational modeling experience. We also describe the contribution of computational models in understanding TERM processes and in advancing the TERM field by providing novel insights.

Lymphocytes have been described to perform different motility patterns such as Brownian random walks, persistent random walks, and Lévy walks. Depending on the conditions, such as confinement or the distribution of target cells, either Brownian or Lévy walks lead to more efficient interaction with the targets. The diversity of these motility patterns may be explained by an adaptive response to the surrounding extracellular matrix (ECM). Indeed, depending on the ECM composition, lymphocytes either display a floating motion without attaching to the ECM, or sliding and stepping motion with respectively continuous or discontinuous attachment to the ECM, or pivoting behaviour with sustained attachment to the ECM. Moreover, on the long term, lymphocytes either perform a persistent random walk or a Brownian-like movement depending on the ECM composition. How the ECM affects cell motility is still incompletely understood. Here, we integrate essential mechanistic details of the lymphocyte-matrix adhesions and lymphocyte intrinsic cytoskeletal induced cell propulsion into a Cellular Potts model (CPM). We show that the combination of de novo cell-matrix adhesion formation, adhesion growth and shrinkage, adhesion rupture, and feedback of adhesions onto cell propulsion recapitulates multiple lymphocyte behaviours, for different lymphocyte subsets and various substrates. With an increasing attachment area and increased adhesion strength, the cells’ speed and persistence decreases. Additionally, the model can predict short-term persistent with long-term subdiffusive motility, showing a pivoting motion. For small adhesion areas, we observe that the spatial distribution of adhesions influences cell motility. Small adhesions at the front allow for more persistent motion than larger clusters at the back, despite a similar total adhesion area. In conclusion, we present an integrated framework to simulate the effects of ECM proteins on cell-matrix adhesion dynamics. The model reveals a sufficient set of principles explaining the plasticity of lymphocyte motility.

Cell-based computational modeling and simulation are becoming invaluable tools in analyzing plant development. In a cell-based simulation model, the inputs are behaviors and dynamics of individual cells and the rules describing responses to signals from adjacent cells. The outputs are the growing tissues, shapes, and cell-differentiation patterns that emerge from the local, chemical, and biomechanical cell-cell interactions. In this updated and extended version of our previous chapter on VirtualLeaf (Merks and Guravage, Methods in Molecular Biology 959, 333–352), we present a step-by-step, practical tutorial for building cell-based simulations of plant development and for analyzing the influence of parameters on simulation outcomes by systematically changing the values of the parameters and analyzing each outcome. We show how to build a model of a growing tissue, a reaction–diffusion system on a growing domain, and an auxin transport model. Moreover, in addition to the previous publication, we demonstrate how to run a Turing system on a regular, rectangular lattice, and how to run parameter sweeps. The aim of VirtualLeaf is to make computational modeling more accessible to experimental plant biologists with relatively little computational background.

Sinds begin deze eeuw kennen we in grote lijnen de volgorde van de letters in het DNA van de mens. Dit humane genoom wordt vaak de ‘blauwdruk van de mens’ genoemd. Ons DNA bepaalt hoe we eruitzien en tot op zekere hoogte zelfs hoe we ons voelen en gedragen. Toch is de werkelijkheid ingewikkelder. Want hoe ontcijfert ons lichaam die DNA-code? Hoe DNA codeert voor een eiwit is weliswaar tot in detail begrepen, maar hoe bepaalt het DNA hoeveel vingers we hebben, hoe onze bloedvaten groeien en hoe ons gezicht eruitziet? Het DNA bepaalt hoe een cel zich gedraagt, maar het kan alleen zeer indirect bepalen hoe cellen zich organiseren tot weefsels, organen en hele orga- nismen. De sleutel ligt bij het begrip van collectief gedrag van cellen.

Aan de hand van klassiek en recent wiskundig, natuurkundig en biologisch onderzoek werd getoond hoe cellen zich kunnen organiseren tot de patronen, vormen en structuren in ons lichaam

Organ laterality refers to the left-right asymmetry in disposition and conformation of internal organs and is established during embryogenesis. The heart is the first organ to display visible left-right asymmetries through its left-sided positioning and rightward looping. Here, we present a new zebrafish loss-of-function allele for tbx5a, which displays defective rightward cardiac looping morphogenesis. By mapping individual cardiomyocyte behavior during cardiac looping, we establish that ventricular and atrial cardiomyocytes rearrange in distinct directions. As a consequence, the cardiac chambers twist around the atrioventricular canal resulting in torsion of the heart tube, which is compromised in tbx5a mutants. Pharmacological treatment and ex vivo culture establishes that the cardiac twisting depends on intrinsic mechanisms and is independent from cardiac growth. Furthermore, genetic experiments indicate that looping requires proper tissue patterning. We conclude that cardiac looping involves twisting of the chambers around the atrioventricular canal, which requires correct tissue patterning by Tbx5a.

Toll-like receptor (TLR) signaling via myeloid differentiation factor 88 protein (MyD88) has been indicated to be involved in the response to wounding. It remains unknown whether the putative role of MyD88 in wounding responses is due to a control of leukocyte cell migration. The aim of this study was to explore in vivo whether TLR2 and MyD88 are involved in modulating neutrophil and macrophage cell migration behavior upon zebrafish larval tail wounding. Live cell imaging of tail-wounded larvae was performed in tlr2 and myd88 mutants and their corresponding wild type siblings. In order to visualize cell migration following tissue damage, we constructed double transgenic lines with fluorescent markers for macrophages and neutrophils in all mutant and sibling zebrafish lines. Three days post fertilization (dpf), tail-wounded larvae were studied using confocal laser scanning microscopy (CLSM) to quantify the number of recruited cells at the wounding area. We found that in both tlr2–/–and myd88–/– groups the recruited neutrophil and macrophage numbers are decreased compared to their wild type sibling controls. Through analyses of neutrophil and macrophage migration patterns, we demonstrated that both tlr2 and myd88 control the migration direction of distant neutrophils upon wounding. Furthermore, in both the tlr2 and the myd88 mutants, macrophages migrated more slowly toward the wound edge. Taken together, our findings show that tlr2 and myd88 are involved in responses to tail wounding by regulating the behavior and speed of leukocyte migration in vivo.

At the origin of multicellularity, cells may have evolved aggregation in response to predation, for functional specialisation or to allow large-scale integration of environmental cues. These group-level properties emerged from the interactions between cells in a group, and determined the selection pressures experienced by these cells. We investigate the evolution of multicellularity with an evolutionary model where cells search for resources by chemotaxis in a shallow, noisy gradient. Cells can evolve their adhesion to others in a periodically changing environment, where a cell’s fitness solely depends on its distance from the gradient source. We show that multicellular aggregates evolve because they perform chemotaxis more efficiently than single cells. Only when the environment changes too frequently, a unicellular state evolves which relies on cell dispersal. Both strategies prevent the invasion of the other through interference competition, creating evolutionary bi-stability. Therefore, collective behaviour can be an emergent selective driver for undifferentiated multicellularity.

Many cells are small and rounded on soft extracellular matrices (ECM), elongated on stiffer ECMs, and flattened on hard ECMs. Cells also migrate up stiffness gradients (durotaxis). Using a hybrid Cellular Potts and finite-element model extended with ODE-based models of focal adhesion (FA) turnover, we show that the full range of cell shape and durotaxis can be explained in unison from dynamics of FAs, in contrast to previous mathematical models. In our 2D cell-shape model, FAs grow due to cell traction forces. Forces develop faster on stiff ECMs, causing FAs to stabilize and, consequently, cells to spread on stiff ECMs. If ECM stress further stabilizes FAs, cells elongate on substrates of intermediate stiffness. We show that durotaxis follows from the same set of assumptions. Our model contributes to the understanding of the basic responses of cells to ECM stiffness, paving the way for future modeling of more complex cell-ECM interactions.

Epithelial branching morphogenesis drives the development of organs such as the lung, salivary gland, kidney and the mammary gland. It involves cell proliferation, cell differentiation and cell migration. An elaborate network of chemical and mechanical signals between the epithelium and the surrounding mesenchymal tissues regulates the formation and growth of branching organs. Surprisingly, when cultured in isolation from mesenchymal tissues, many epithelial tissues retain the ability to exhibit branching morphogenesis even in the absence of proliferation. In this work, we propose a simple, experimentally plausible mechanism that can drive branching morphogenesis in the absence of proliferation and cross-talk with the surrounding mesenchymal tissue. The assumptions of our mathematical model derive from in vitro observations of the behaviour of mammary epithelial cells. These data show that autocrine secretion of the growth factor TGFβ1 inhibits the formation of cell protrusions, leading to curvature-dependent inhibition of sprouting. Our hybrid cellular Potts and partial-differential equation model correctly reproduces the experimentally observed tissue-geometry-dependent determination of the sites of branching, and it suffices for the formation of self-avoiding branching structures in the absence and also in the presence of cell proliferation.
This article is part of the theme issue ‘Multi-scale analysis and modelling of collective migration in biological systems’.

“Cellular Connections” shows a computer simulation of the growth of blood vessels. Endothelial cells, the building blocks of blood vessels, collectively form networks of blood vessels, much like ants work together to form their nests. In the center of the image, the cells are sufficiently close together such that they feel one another and manage to interconnect. The cells at the periphery are too far away and wander around aimlessly.

The formation of blood vessels plays a pivotal role in tumour growth. “Pulled in line” shows a simulation of how a tumour could attract blood vessels by pulling on the extracellular matrix. Many cell types in our body are able to apply forces on their surroundings. The gel-like structure surrounding cells and tissues is called the extracellular matrix. It becomes stressed due to the pulling forces of cells. Because cells can feel and respond to these stresses, cells can communicate their position to other cells.

Vertebrates are characterized by their segmented structure, first visible in the somites that form along the embryonic body axis. Somites are the predecessors of the vertebrae, ribs, muscles and the skin and impose a segmented organization on the peripheral nervous system. The variability in somite number between species has presumably evolved by genetic rearrangements, leading to changes in the ratio of the segmentation clock rate to the developmental growth rate. However, it is known that physical cues, like temperature, salinity or light conditions, can modify the vertebrate body plan and change the vertebral number. Here we show that mechanical stretching, another physical cue, can induce the formation of additional somites in the developing chicken embryo. Stretching of live chick embryos and the resulting deformation of somites induces a slow cellular reorganization of somites to form two or more well-shaped and stable daughter somites. Mesenchymal cells from the somite core thereby undergo mesenchymal-to-epithelial transitions (MET), thus meeting the geometrical demand for more border cells. Our simulations, using a Cellular Potts Model of somite remodeling, suggest that this MET occurs through lateral induction by existing epithelial cells. Our results strengthen the idea that somitic mesoderm self-organizes, and show that it is phenotypically plastic under variations in the mechanical environment. These somite qualities could play out as a selective advantage by preventing the formation of transitional vertebrae and give rise to another possibility how the vertebrate body axis might have evolved towards different vertebral numbers, next to the previously proposed genetic rearrangements.

Recent experimental studies have demonstrated that cellular motion can be directed by topographical gradients, such as those resulting from spatial variations in the features of a micropatterned substrate. This phenomenon, known as topotaxis, is especially prominent among cells persistently crawling within a spatially varying distribution of cell-sized obstacles. In this article we introduce a toy model of topotaxis based on active Brownian particles constrained to move in a lattice of obstacles, with space-dependent lattice spacing. Using numerical simulations and analytical arguments, we demonstrate that topographical gradients introduce a spatial modulation of the particles' persistence, leading to directed motion toward regions of higher persistence. Our results demonstrate that persistent motion alone is sufficient to drive topotaxis and could serve as a starting point for more detailed studies on self-propelled particles and cells.

Recent experiments on monolayers of spindle-like cells plated on adhesive stripe-shaped domains have provided a convincing demonstration that certain types of collective phenomena in epithelia are well described by active nematic hydrodynamics. While recovering some of the hallmark predictions of this framework, however, these experiments have also revealed a number of unexpected features that could be ascribed to the existence of chirality over length scales larger than the typical size of a cell. In this article we elaborate on the microscopic origin of chiral stresses in nematic cell monolayers and investigate how chirality affects the motion of topological defects, as well as the collective motion in stripe-shaped domains. We find that chirality introduces a characteristic asymmetry in the collective cellular flow, from which the ratio between chiral and non-chiral active stresses can be inferred by particle-image-velocimetry measurements. Furthermore, we find that chirality changes the nature of the spontaneous flow transition under confinement and that, for specific anchoring conditions, the latter has the structure of an imperfect pitchfork bifurcation.

This Special Issue consists of contributions from participants of three workshops with similar focus held in 2016–17:

• “Modelling of Tissue Growth and Form” held from March 6 to March 10, 2017, at the NSF Mathematical Biology Institute (MBI), Columbus, OH, USA,
• “Multi-scale Modeling of Complex Systems in Developmental and Plant Biology” held on December 15, 2017, at the Interdisciplinary Center for Quantitative Modeling in Biology, University of California, Riverside, CA, USA,
• “Computing a Tissue: Modeling Multicellular Systems” at the 15th European Conference on Computational Biology held from September 3 to September 7, 2016, at The Hague, Netherlands.

We investigate the mechanical interplay between the spatial organization of the actin cytoskeleton and the shape of animal cells adhering on micropillar arrays. Using a combination of analytical work, computer simulations and in vitro experiments, we demonstrate that the orientation of the stress fibers strongly influences the geometry of the cell edge. In the presence of a uniformly aligned cytoskeleton, the cell edge can be well approximated by elliptical arcs, whose eccentricity reflects the degree of anisotropy of the cell’s internal stresses. Upon modeling the actin cytoskeleton as a nematic liquid crystal, we further show that the geometry of the cell edge feeds back on the organization of the stress fibers by altering the length scale at which these are confined. This feedback mechanism is controlled by a dimensionless number, the anchoring number, representing the relative weight of surface- anchoring and bulk-aligning torques. Our model allows to predict both cellular shape and the internal structure of the actin cytoskeleton and is in good quantitative agreement with experiments on fibroblastoid (GDβ1, GDβ3) and epithelioid (GEβ1, GEβ3) cells.

Zebrafish is a useful modeling organism for the study of vertebrate development, immune response and metabolism. Metabolic studies can be aided by mathematical reconstructions of the metabolic network of the zebrafish. These list the substrates and products of all biochemical reactions that occur in the zebrafish. Mathematical techniques such as flux-balance analysis then make it possible to predict the possible metabolic flux distributions that optimize, for example, the turn-over of food into biomass. The only available genome-scale reconstruction of zebrafish metabolism is ZebraGEM (Bekaert, PLOS ONE 2012). Here we present ZebraGEM 2.0, an updated and validated version of ZebraGEM. ZebraGEM 2.0 is extended with gene-protein-reactions associations (GPRs) that are required to integrate genetic data with the metabolic model. To demonstrate the use of these GPRs we performed an in silico genetic screening for knock-outs of metabolic genes, and validated the results against published in vivo genetic knockout and knockdown screenings. Among the single-knockout simulations we identified 74 essential genes, whose knock-out stopped growth completely. Among these, 11 genes are known have an abnormal knock-out or knock-down phenotype in vivo (partial), and 41 have human homologs associated with metabolic diseases. We also added the oxidative phosphorylation pathway, which was unavailable in the published version of ZebraGEM. The updated model performs better than the original model on a predetermined list of metabolic functions. We also determined a minimal feed composition. The oxidative phosphorylation pathways was validated by comparing with published experiments in which key components of the oxidative phosphorylation pathway were pharmacologically inhibited. To test the utility of ZebraGEM2.0 for obtaining new results, we integrated gene expression data from control and Mycobacterium marinum-infected zebrafish larvae. The resulting model predicts impeded growth and altered histidine metabolism in the infected larvae.

Cell-based, mathematical modeling of collective cell behavior has become a prominent tool in developmental biology. Cell-based models represent individual cells as single particles or as sets of interconnected particles, and predict the collective cell behavior that follows from a set of interaction rules. In particular, vertex-based models are a popular tool for studying the mechanics of confluent, epithelial cell layers. They represent the junctions between three (or sometimes more) cells in confluent tissues as point particles, connected using structural elements that represent the cell boundaries. A disadvantage of these models is that cell-cell interfaces are represented as straight lines. This is a suitable simplification for epithelial tissues, where the interfaces are typically under tension, but this simplification may not be appropriate for mesenchymal tissues or tissues that are under compression, such that the cell-cell boundaries can buckle. In this paper we introduce a variant of VMs in which this and two other limitations of VMs have been resolved. The new model can also be seen as on off-the-lattice generalization of the Cellular Potts Model. It is an extension of the open-source package VirtualLeaf, which was initially developed to simulate plant tissue morphogenesis where cells do not move relative to one another. The present extension of VirtualLeaf introduces a new rule for cell-cell shear or sliding, from which T1 and T2 transitions emerge naturally, allowing application of VirtualLeaf to problems of animal development. We show that the updated VirtualLeaf yields different results than the traditional vertex-based models for differential-adhesion-driven cell sorting and for the neighborhood topology of soft cellular networks

We investigate the geometrical and mechanical properties of adherent cells characterized by a highly anisotropic actin cytoskeleton. Using a combination of theoretical work and experiments on micropillar arrays, we demonstrate that the shape of the cell edge is accurately described by elliptical arcs, whose eccentricity expresses the degree of anisotropy of the internal cell stresses. This results in a spatially varying tension along the cell edge, that significantly affects the traction forces exerted by the cell on the substrate. Our work highlights the strong interplay between cell mechanics and geometry and paves the way towards the reconstruction of cellular forces from geometrical data

In experimental assays of angiogenesis in three-dimensional fibrin matrices, a temporary scaffold formed during wound healing, the type and composition of fibrin impacts the level of sprouting. More sprouts form on high molecular weight (HMW) than on low molecular weight (LMW) fibrin. It is unclear what mechanisms regulate the number and the positions of the vascular-like structures in cell cultures. To address this question, we propose a mechanistic simulation model of endothelial cell migration and fibrin proteolysis by the plasmin system. The model is a hybrid, cell-based and continuum, computational model based on the cellular Potts model and sets of partial-differential equations. Based on the model results, we propose that a positive feedback mechanism between uPAR, plasmin and transforming growth factor β1 (TGFβ1) selects cells in the monolayer for matrix invasion. Invading cells releases TGFβ1 from the extracellular matrix through plasmin-mediated fibrin degradation. The activated TGFβ1 further stimulates fibrin degradation and keeps proteolysis active as the sprout invades the fibrin matrix. The binding capacity for TGFβ1 of LMW is reduced relative to that of HMW. This leads to reduced activation of proteolysis and, consequently, reduced cell ingrowth in LMW fibrin compared to HMW fibrin. Thus our model predicts that endothelial cells in LMW fibrin matrices compared to HMW matrices show reduced sprouting due to a lower bio-availability of TGFβ1. [ open access at PLOS Computational Biology ]

The cellular Potts model (CPM, a.k.a. Glazier–Graner–Hogeweg or GGH model) is a somewhat liberal extension of probabilistic cellular automata. The model is derived from the Ising and Potts models and represents biological cells as domains of CA-sites of the same state. A Hamiltonian energy is used to describe the balance of forces that the biological cells apply onto one another and their local environment. A Metropolis algorithm iteratively copies the state from one site into one of the adjacent sites, thus shifting the domain interfaces and moving the biological cells along the lattice. The approach is commonly used in applications of developmental biology, where the CPM often interacts with systems of ordinary-differential equations that model the intracellular chemical kinetics and partial-differential equations that model the extracellular chemical signal dynamics to constitute a hybrid and multiscale description of the biological system. In this chapter we will introduce the cellular Potts model and discuss its use in developmental biology, focusing on the development of blood vessels, a process called vascular morphogenesis. We will start by introducing a range of models focusing on uncovering the basic mechanisms of vascular morphogenesis: network formation and sprouting and then show how these models are extended with models of intracellular regulation and with interactions with the extracellular micro-environment. We then briefly review the integration of models of vascular morphogenesis in several examples of organ development in health and disease, including development, cancer, and age-related macular degeneration. We end by discussing the computational efficiency of the CPM and the available strategies for the validation of CPM-based simulation models.

In January 1970, computer scientist Leo Geurts walked into Swart Gallery in Amsterdam, The Netherlands, to see the solo exhibition by Dutch artist Peter Struycken (The Netherlands, 1939). He was struck by Struycken’s black and white works “Computerstructuren” (1969), which were painted after grid patterns generated by algorithms. Geurts assumed that they must have been produced using cellular automata. He started working with Lambert Meertens at Mathematisch Centrum (now CWI) in Amsterdam to make a similar work.

Cancer is a disease of cellular regulation, often initiated by genetic mutation within cells, and leading to a heterogeneous cell population within tissues. In the competition for nutrients and growth space within the tumors the phenotype of each cell determines its success. Selection in this process is imposed by both the microenvironment (neighboring cells, extracellular matrix, and diffusing substances), and the whole of the organism through for example the blood supply. In this view, the development of tumor cells is in close interaction with their increasingly changing environment: the more cells can change, the more their environment will change. Furthermore, instabilities are also introduced on the organism level: blood supply can be blocked by increased tissue pressure or the tortuousity of the tumor-neovascular vessels. This coupling between cell, microenvironment, and organism results in behavior that is hard to predict. Here we introduce a cell-based computational model to study the effect of blood flow obstruction on the micro-evolution of cells within a tumorous tissue. We demonstrate that stages of tumor development emerge naturally, without the need for sequential mutation of specific genes. Secondly, we show that instabilities in blood supply can impact the overall development of tumors and lead to the extinction of the dominant aggressive phenotype, showing a clear distinction between the fitness at the cell level and fitness at the population level. This provides new insights into potential side effects of recent tumor vasculature renormalization approaches

Background: The human gut contains approximately 1.0e+14 bacteria, belonging to hundreds of different species. Together, these microbial species form a complex food web that can break down food sources that our own digestive enzymes cannot handle, including complex polysaccharides, producing short chain fatty acids and additional metabolites, e.g., vitamin K. The diversity of microbial diversity is important for colonic health: Changes in the composition of the microbiota have been associated with inflammatory bowel disease, diabetes, obestity and Crohn's disease, and make the microbiota more vulnerable to infestation by harmful species, e.g., Clostridium difficile. To get a grip on the controlling factors of microbial diversity in the gut, we here propose a multi-scale, spatiotemporal dynamic flux-balance analysis model to study the emergence of metabolic diversity in a spatial gut-like, tubular environment. The model features genome-scale metabolic models of microbial populations, resource sharing via extracellular metabolites, and spatial population dynamics and evolution.
Results: In this model, cross-feeding interactions emerge readily, despite the species' ability to metabolize sugars autonomously. Interestingly, the community requires cross-feeding for producing a realistic set of short-chain fatty acids from an input of glucose, If we let the composition of the microbial subpopulations change during invasion of adjacent space, a complex and stratifed microbiota evolves, with subspecies specializing on cross-feeding interactions via a mechanism of compensated trait loss. The microbial diversity and stratification collapse if the flux through the gut is enhanced to mimic diarrhea.
Conclusions: In conclusion, this in silico model is a helpful tool in systems biology to predict and explain the controlling factors of microbial diversity in the gut. It can be extended to include, e.g., complex food source, and host-microbiota interactions via the gut wall.

Mathematical modeling is an essential approach for the understanding of complex multicellular behaviors in tissue morphogenesis. Here, we review the cellular Potts model (CPM; also known as the Glazier-Graner-Hogeweg model), an effective computational modeling framework. We discuss its usability for modeling complex developmental phenomena by examining four fundamental examples of tissue morphogenesis: (1) cell sorting, (2) cyst formation, (3) tube morphogenesis in kidney development, and (4) blood vessel formation. The review provides an introduction for biologists for starting simulation analysis using the CPM framework.

During animal development and homeostasis, the structure of tissues, including muscles, blood vessels, and connective tissues, adapts to mechanical strains in the extracellular matrix (ECM). These strains originate from the differential growth of tissues or forces due to muscle contraction or gravity. Here we show using a computational model that by amplifying local strain cues, active cell contractility can facilitate and accelerate the reorientation of single cells to static strains. At the collective cell level, the model simulations show that active cell contractility can facilitate the formation of strings along the orien- tation of stretch. The computational model is based on a hybrid cellular Potts and finite-element simulation framework describing a mechanical cell-substrate feedback, where: 1) cells apply forces on the ECM, such that 2) local strains are generated in the ECM and 3) cells preferentially extend protrusions along the strain orientation. In accordance with experimental observations, simulated cells align and form stringlike structures parallel to static uniaxial stretch. Our model simulations predict that the magnitude of the uniaxial stretch and the strength of the contractile forces regulate a gradual transition between stringlike patterns and vascular networklike patterns. Our simulations also suggest that at high population densities, less cell cohesion promotes string formation

Exchanging and understanding scientific data and their context represents a significant barrier to advancing research, especially with respect to information siloing. Maintaining information provenance and providing data curation and quality control help overcome common concerns and barriers to the effective sharing of scientific data. To address these problems in and the unique challenges of multicellular systems, we assembled a panel composed of investigators from several disciplines to create the MultiCellular Data Standard (MultiCellDS) with a use-case driven development process. The standard includes (1) digital cell lines, which are analogous to traditional biological cell lines, to record metadata, cellular microenvironment, and cellular phenotype variables of a biological cell line, (2) digital snapshots to consistently record simulation, experimental, and clinical data for multicellular systems, and (3) collections that can logically group digital cell lines and snapshots. We have created a MultiCellular DataBase (MultiCellDB) to store digital snapshots and the 200+ digital cell lines we have generated. MultiCellDS, by having a fixed standard, enables discoverability, extensibility, maintainability, searchability, and sustainability of data, creating biological applicability and clinical utility that permits us to identify upcoming challenges to uplift biology and strategies and therapies for improving human health.

Angiogenesis involves the formation of new blood vessels by sprouting or splitting of existing blood vessels. During sprouting, a highly motile type of endothelial cell, called the tip cell, migrates from the blood vessels followed by stalk cells, an endothelial cell type that forms the body of the sprout. In vitro models and computational models can recapitulate much of the phenomenology of angiogenesis in absence of tip and stalk cell differentiation. Therefore it is unclear how the presence of tip cells contributes to angiogenesis. To get more insight into how tip cells contribute to angiogenesis, we extended an existing computational model of vascular network formation based on the cellular Potts model with tip and stalk differentiation, without making a priori assumptions about the specific rules that tip cells follow. We then screened a range of model variants, looking for rules that make tip cells (a) move to the sprout tip, and (b) change the morphology of the angiogenic networks. The screening predicted that if tip cells respond less effectively to an endothelial chemoattractant than stalk cells, they move to the tips of the sprouts, which impacts the morphology of the networks. A comparison of this model prediction with genes expressed differentially in tip and stalk cells revealed that the endothelial chemoattractant Apelin and its receptor APJ may match the model prediction. To test the model prediction we inhibited Apelin signaling in our model and in an in vitro model of angiogenic sprouting, and found that in both cases inhibition of Apelin or of its receptor APJ reduces sprouting. Based on the prediction of the computational model, we propose that the differential expression of Apelin and APJ yields a "self-generated" gradient mechanisms that accelerates the extension of the sprout.

Shapes of the cell have fascinated our curiosity since the discovery of the cell by Robert Hooke in 16651 and by Anton van Leeuwenhoek slightly later2 using the state-of-the-art microscopes of the time. In medicine, cell shapes have long been exploited to diagnose disease types and conditions, such as cancer types and malignant vs. benign tumors, respectively. (...)

During vessel sprouting, endothelial cells (ECs) dynamically rearrange positions in the sprout to compete for the tip position. We recently identified a key role for the glycolytic activator PFKFB3 in vessel sprouting by regulating cytoskeleton remodelling, migration and tip cell competitiveness. It is, however, unknown how glycolysis regulates EC rearrangement during vessel sprouting. Here we report that computational simulations, validated by experimentation, predict that glycolytic production of ATP drives EC rearrangement by promoting filopodia formation and reducing intercellular adhesion. Notably, the simulations correctly predicted that blocking PFKFB3 normalizes the disturbed EC rearrangement in high VEGF conditions, as occurs during pathological angiogenesis. This interdisciplinary study integrates EC metabolism in vessel sprouting, yielding mechanistic insight in the control of vessel sprouting by glycolysis, and suggesting anti-glycolytic therapy for vessel normalization in cancer and non-malignant diseases.

Cell shape influences function, and the current model suggests that such shape effect is transient. However, cells dynamically change their shapes, thus, the critical question is whether shape information remains influential on future cell function even after the original shape is lost. We address this question by integrating experimental and computational approaches. Quantitative live imaging of asymmetric cell-fate decision-making and their live shape manipulation demonstrates that cellular eccentricity of progenitor cell indeed biases stochastic fate decisions of daughter cells despite mitotic rounding. Modelling and simulation indicates that polarized localization of Delta protein instructs by the progenitor eccentricity is an origin of the bias. Simulation with varying parameters predicts that diffusion rate and abundance of Delta molecules quantitatively influence the bias. These predictions are experimentally validated by physical and genetic methods, showing that cells exploit a mechanism reported herein to influence their future fates based on their past shape despite dynamic shape changes.

The self-organization of endothelial cells into blood vessel networks and sprouts can be studied using computational, cell-based models. These take as input the behavior of individual, endothelial cells, as observed in experiments, and gives as output the resulting, collective behavior, i.e. the formation of shapes and tissue structures. Many cell-based models ignore the extracellular matrix, i.e., the fibrous or homogeneous materials that surround cells and gives tissue structural support. In this extended abstract, we highlight two approaches that we have taken to explore the role of the extracellular matrix in our cellular Potts models of blood vessel formation (angiogenesis): first we discuss a model considering chemical endothelial cell-matrix interactions, then we discuss a model that include mechanical cell-matrix interactions. We end by discussing some potential new directions.

A cell-based model is a simulation model that predicts collective behavior of cell-clusters from the behavior and interactions of individual cells.  The inputs to a cell-based model are cell behaviors as observed in experiments or deriving from single cell models, including the cellular responses to cues from the micro-environment. The cell behaviors are encoded in a set of biologically plausible rules that the simulated cells will follow. The outputs of a cell-based model are the patterns and behaviors that follow indirectly from the cell behaviors and the cellular interactions. Cell-based models resemble agent-based models, but typically contain more biophysically-detailed descriptions of the individual cells.

During angiogenesis, endothelial cells compete for the tip position during angiogenesis: a phenomenon named tip cell overtaking. It is still unclear to what extent tip cell overtaking is a side effect of sprouting or to what extent a biological function. To address this question, we studied tip cell overtaking in two existing cellular Potts models of angiogenic sprouting. In these models angiogenic sprouting-like behavior emerges from a small set of plausible cell behaviors and the endothelial cells spontaneously migrate forwards and backwards within sprouts, suggesting that tip cell overtaking might occur as a side effect of sprouting. In accordance with experimental observations, in our simulations the cells' tendency to occupy the tip position can be regulated when two cell lines with different levels of Vegfr2 expression are contributing to sprouting (mosaic sprouting assay), where cell behavior is regulated by a simple VEGF-Dll4-Notch signaling network. Our modeling results suggest that tip cell overtaking occurs spontaneously due to the stochastic motion of cells during sprouting. Thus, tip cell overtaking and sprouting dynamics may be interdependent and should be studied and interpreted in combination. VEGF-Dll4-Notch can regulate the ability of cells to occupy the tip cell position, but only when cells in the simulation strongly differ in their levels of Vegfr2. We propose that VEGF-Dll4-Notch signaling might not regulate which cell ends up at the tip, but assures that the cell that randomly ends up at the tip position acquires the tip cell phenotype.

Morphogenesis is a developmental process in which cells organize into shapes and patterns. Complex, multi-factorial models are commonly used to study morphogenesis. It is difficult to understand the relation between the uncertainty in the input and the output of such `black-box' models, giving rise to the need for sensitivity analysis tools. In this paper, we introduce a workflow for a global sensitivity analysis approach to study the impact of single parameters and the interactions between them on the output of morphogenesis models. To demonstrate the workflow, we used a published, well-studied model of vascular morphogenesis. The parameters of the model represent cell properties and behaviors that drive the mechanisms of angiogenic sprouting. The global sensitivity analysis correctly identified the dominant parameters in the model, consistent with previous studies. Additionally, the analysis provides information on the relative impact of single parameters and of interactions between them. The uncertainty in the output of the model was largely caused by single parameters. The parameter interactions, although of low impact, provided new insights in the mechanisms of \emph{in silico} sprouting. Finally, the analysis indicated that the model could be reduced by one parameter. We propose global sensitivity analysis as an alternative approach to study and validate the mechanisms of morphogenesis. Comparison of the ranking of the impact of the model parameters to knowledge derived from experimental data and validation of manipulation experiments can help to falsify models and to find the operand mechanisms in morphogenesis. The workflow is applicable to all `black-box' models, including high-throughput in vitro models in which an output measure is affected by a set of experimental perturbations.

Computational modelling is helpful for elucidating the cellular mechanisms driving biological morphogenesis. Previous simulation studies of blood vessel growth based on the Cellular Potts model (CPM) proposed that elongated, adhesive or mutually attractive endothelial cells suffice for the formation of blood vessel sprouts and vascular networks. Because each mathematical representation of a model introduces potential artifacts, it is important that model results are reproduced using alternative modelling paradigms. Here, we present a lattice-free, particle-based simulation of the cell elongation model of vasculogenesis. The new, particle-based simulations confirm the results obtained from the previous Cellular Potts simulations. Furthermore, our current findings suggest that the emergence of order is possible with the application of a high enough attractive force or, alternatively, a longer attraction radius. The methodology will be applicable to a range of problems in morphogenesis and noisy particle aggregation in which cell shape is a key determining factor

How does the genetic information encoded in the DNA direct the growth and form of multicellular organisms? The genome directs a cell`s biophysical properties and its response to signals from adjacent cells. To analyse how these cell behaviours drive embryogenesis, insights into the collective behaviour of multicellular aggregates is crucial. This article describes computational approaches to the study of collective cell behaviour. Four examples (cell sorting, angiogenesis, somitogenesis, and root development) illustrate how insights into collective cell behaviour are incorporated into experimental studies to unravel the mechanisms of development.

In angiogenesis, the process in which blood vessel sprouts grow out from a pre-existing vascular network, the so-called endothelial tip cells play an essential role. Tip cells are the leading cells of the sprouts; they guide following endothelial cells and sense their environment for guidance cues. Because of this essential role, the tip cells are a potential therapeutic target for anti-angiogenic therapies, which need to be developed for dis- eases such as cancer and major eye diseases. The potential of anti-tip cell therapies is now widely recognised, and the surge in research this has caused has led to improved insights in the func- tion and regulation of tip cells, as well as the development of novel in vitro and in silico models. These new models in particular will help under- stand essential mechanisms in tip cell biology and may eventually lead to new or improved therapies to prevent blindness or cancer spread.

Excessive migration and proliferation of smooth muscle cells has been observed as a major factor contributing to the development of in-stent restenosis after coronary stenting. Building upon the results from in-vivo experiments, we formulated a hypothesis that the speed of the initial tissue re-growth response is determined by the early migration of smooth muscle cells from the injured intima. To test this hypothesis, a Cellular Potts model of the stented artery is developed where stent struts were deployed at different depths into the tissue. An extreme scenario with a ruptured internal elastic lamina was also considered to study the role of severe injury on the tissue re-growth. Based on the outcomes, we hypothesize that a deeper stent deployment results in on average larger fenestrae in the elastic lamina, allowing easier migration of smooth muscle cells into the lumen. The data also suggest that growth of the neointimal lesions due to smooth muscle cells proliferation is strongly dependent on the initial number of migrated cells, which form an initial condition for the later phase of the vascular repair. This mechanism could explain the in-vivo observation that the initial rate of neointima formation and injury score are strongly correlated.

Hoe beschrijft de informatie in het DNA de groei en vorm van dieren of planten? De wiskunde helpt de biologie om dit complexe biologische mechanisme te doorgronden. Nieuwe experimentele technieken hebben de laatste decennia grote hoeveelheden gegevens opgeleverd over de moleculen van het leven: Big Data. Maar echt begrip van de biologische mechanismen achter groei en vorm dreigt juist door Big Data moeilijker te worden: we dreigen het overzicht kwijt te raken.

Tissue patterning is established by extracellular growth factors or morphogens. Although different theoretical models explaining specific patterns have been proposed, our understanding of tissue pattern establishment in vivo remains limited. In many animal species, left-right patterning is governed by a reac- tion-diffusion system relying on the different diffusivity of an activator, Nodal, and an inhibitor, Lefty. In a genetic screen, we identified a zebrafish loss- of-function mutant for the proprotein convertase FurinA. Embryological and biochemical experiments demonstrate that cleavage of the Nodal-related Spaw proprotein into a mature form by FurinA is required for Spaw gradient formation and activation of Nodal signaling. We demonstrate that FurinA is required cell-autonomously for the long-range sig- naling activity of Spaw and no other Nodal-related factors. Combined in silico and in vivo approaches support a model in which FurinA controls the sig- naling range of Spaw by cleaving its proprotein into a mature, extracellular form, consequently regulating left-right patterning.

Hoe bepaalt het DNA de groei en vorm van plant en dier? Wat als er een fout zit in het DNA, een mutatie? Zulke vragen houden mij en mijn onderzoeksgroep bezig op het Centrum Wiskunde & Informatica (CWI) in Amsterdam en op het Mathematisch Instituut in Leiden.

Big data research in Life Sciences typically focuses on big molecular datasets of protein structures, DNA sequences, gene expression, proteomics and metabolomics. Now, however, new developments in three-dimensional imaging and microscopy have started to deliver big datasets of cell behaviours during embryonic development including cell trajectories and shapes and patterns of gene activity from every position in the embryo. This surge of multicellular and multi-scale biological data poses exciting new challenges for the application of ICT and applied mathematics in this field.

Roeland M.H. Merks.  The Next Boom of Big Data in Biology: Multicellular Datasets.

Angiogenesis is the process by which new blood vessels develop by splitting of or by sprouting from existing vessels. In sprouting angiogenesis vessels branch out and connect with other sprouts to form a new network. This process involves both the endothelial cells, which make up the inner lining of a vessel, and the perivascular cells, which surround the vessel. The collective behavior of these cells results in the formation of sprouts and eventually vascular networks. The cells involved in angiogenesis differ in shape and behavior, which affects their collective behavior. Furthermore, the cells also affect one another via diffusive and membrane bound signaling molecules. In this thesis we aim to understand how interactions between multiple cell-types exhibiting subtle differences in behavior change the resulting collective angiogenic sprouting. To this end, we developed cell-based, computational models of angiogenesis, based on the cellular Potts model. The inputs of these models are the observed or hypothesized behavior of individual cells and the output is the resulting collective cell behavior: e.g., the formation of angiogenic sprouts or vascular networks. By assigning different behavior to a subset of the cells, these models can be used to study the interplay between cell types exhibiting different behavior.

In vitro cultures of endothelial cells are a widely used model system of the collective behavior of endothelial cells during vasculogenesis and angiogenesis. When seeded in an extracellular matrix, endothelial cells can form blood vessel-like structures, including vascular networks and sprouts. Endothelial morphogenesis depends on a large number of chemical and mechanical factors, including the compliancy of the extracellular matrix, the available growth factors, the adhesion of cells to the extracellular matrix, cell-cell signaling, etc. Although various computational models have been proposed to explain the role of each of these biochemical and biomechanical effects, the understanding of the mechanisms underlying in vitro angiogenesis is still incomplete. Most explanations focus on predicting the whole vascular network or sprout from the underlying cell behavior, and do not check if the same model also correctly captures the intermediate scale: the pairwise cell-cell interactions or single cell responses to ECM mechanics. Here we show, using a hybrid cellular Potts and finite element computational model,  that a single set of biologically plausible rules describing (a) the contractile forces that endothelial cells exert on the ECM, (b) the resulting strains in the extracellular matrix, and (c) the cellular response to the strains, suffices for reproducing the behavior of individual endothelial cells and the interactions of endothelial cell pairs in compliant matrices. With the same set of rules, the model also reproduces network formation from scattered cells, and sprouting from endothelial spheroids. Combining the present mechanical model with aspects of previously proposed mechanical and chemical models may lead to a more complete understanding of in vitro angiogenesis.

Computational modeling has become a widely used tool for unraveling the mechanisms of higher-level cooperative cell behavior during vascular morphogenesis. However, experimenting with published simulation models or adding new assumptions to those models can be daunting for novice and even for experienced computational scientists. Here, we present a step-by-step, practical tutorial for building cell-based simulations of vascular morphogenesis using the Tissue Simulation Toolkit (TST). The TST is a freely available, open-source C++ library for developing simulations with the two-dimensional Cellular Potts model, a stochastic, agent-based framework to simulate collective cell behavior. We will show the basic use of the TST to simulate and experiment with published simulations of vascular network formation. Then, we will present step-by-step instructions and explanations for building a recent simulation model of tumor angiogenesis. Demonstrated mechanisms include cell-cell adhesion, chemotaxis, cell elongation, haptotaxis, and haptokinesis

Computational, cell-based models, such as the cellular Potts model (CPM) have become a widely-used tool to study tissue formation. Most  cell-based models mimic the physical properties of cells and their  dynamic behavior, and generate images of the tissue that the cells form  due to their collective behavior. Due to these intuitive parameters and output, cell-based models are often evaluated visually and the parameters are fine-tuned by hand. To get better insight into how in a cell-based model the microscopic scale (e.g., cell behavior, secreted molecular signals, and cell-ECM interactions) determines the macroscopic scale, we need to generate morphospaces and perform parameter sweeps, involving large numbers of individual simulations. This chapter describes a protocol and presents a set of scripts for automatically setting up, running and evaluating large-scale parameter sweeps of cell-based models. We demonstrate the use of the protocol using a recent cellular Potts model of blood vessel formation model implemented in CompuCell3D. We show the versatility of the protocol by adapting it to an alternative cell-based modeling framework, VirtualLeaf.

A key step in blood vessel development (angiogenesis) is lumen formation: the hollowing of vessels for blood perfusion. Two alternative lumen formation mechanisms are suggested to function in different types of blood vessels. The vacuolation mechanism is suggested for lumen formation in small vessels by coalescence of intracellular vacuoles, a view that was extended to extracellular lumen formation by exocytosis of vacuoles. The cell-cell repulsion mechanism is suggested to initiate extracellular lumen formation in large vessels by active repulsion of adjacent cells, and active cell shape changes extend the lumen.  We used an agent-based computer model, based on the Cellular Potts Model, to compare and study both mechanisms separately and combined. An extensive sensitivity analysis shows that each of the mechanisms on its own can produce lumens in a narrow region of parameter space. However, combining both mechanisms makes lumen formation much more robust to the values of the parameters, suggesting that the mechanisms may work synergistically and operate in parallel, rather than in different vessel types.

Despite a growing wealth of available molecular data, the growth of tumors, invasion of tumors into healthy tissue, and response of tumors to therapies are still poorly understood. Although genetic mutations are in general the first step in the development of a cancer,  for the mutated cell to persist in a tissue, it must compete against the other, healthy or diseased cells, for example by becoming more motile, adhesive, or multiplying faster. Thus, the cellular phenotype determines the success of a cancer cell in competition with its neighbors, irrespective of the genetic mutations or physiological alterations that gave rise to the altered phenotype.

What phenotypes can make a cell "successful" in an environment of healthy and cancerous cells, and how?  A widely-used tool for getting more insight into that question is cell-based modeling. Cell based models constitute a class of computational, agent-based models that mimic biophysical and molecular interactions between cells. One of the most widely used cell-based modeling formalisms is the cellular Potts model (CPM),  a lattice-based, multi particle cell-based modeling approach. The CPM has become the most mature and accessible method for modeling mechanisms of multicellular processes ranging from cell sorting through gastrulation and angiogenesis, to modeling tumor progression. The CPM accounts for biophysical cellular properties, including cell proliferation, cell motility, and cell adhesion, which play a key role in cancer.  Multiscale models are constructed by extending the agents with intracellular processes including metabolism, growth, and signaling. Here we review the use of the CPM for modeling tumor growth, tumor invasion, and tumor progression. We argue that the accessibility and flexibility of the CPM, and its accurate, yet coarse-grained and computationally efficient representation of cell- and tissue biophysics, make the CPM the method of choice for modeling cellular processes in tumor development.

Angiogenesis, the formation of new blood vessels sprouting from existing ones, occurs in several situations like wound healing, tissue remodeling and near growing tumors. Under hypoxic conditions tumor cells secrete growth factors, including VEGF. VEGF activates endothelial cells (ECs) in nearby vessels, leading to the migration of ECs out of the vessel and the formation of growing sprouts. A key process in angiogenesis is cellular self-organization, and previous modeling studies have identified mechanisms for producing networks and sprouts. Most theoretical studies of cellular self-organization during angiogenesis have ignored the interactions of ECs with the extra-cellular matrix (ECM), the jelly or hard materials that cells live in. Apart from providing structural support to cells, the ECM may play a key role in the coordination of cellular motility during angiogenesis. For example, by modifying the ECM, ECs can affect the motility of other ECs, long after they have left. Here we present an explorative study of the cellular self-organization resulting from such ECM-coordinated cell migration. We show that a set of biologically-motivated, cell behavioral rules, including chemotaxis, haptotaxis, haptokinesis, and ECM-guided proliferation suffice for forming sprouts and branching vascular trees.

During embryonic development cardiac valves arise at specific regions in  the cardiac endothelium that swell up due to enhanced extracellular  matrix production (so called endocardial cushions). An important  extracellular matrix component that is produced by the endocardial cells  is the glycosaminoglycan hyaluronan. A deficiency in hyaluronan  synthesis results in a failure to form endocardial cushions and a loss  of their cellularization by a process called endothelial-to-mesenchymal  transformation. Expression of the major hyaluronan synthase Has2 is  under the influence of both positive and negative regulators. MicroRNA  dependent degradation of Has2 is required to control extracellular  hyaluronan levels and thereby the size of the endocardial cushions. In  this article we review the current literature on hyaluronan synthesis  during cardiac valve formation and propose that a balanced activity of  both positive and negative regulators is required to maintain the  critical homeostasis of hyaluronan levels in the extracellular matrix  and thereby the size of the endocardial cushions.  The activating and  inhibitory interactions between microRNA-23, Has2 and hyaluronan are  reminiscent of a reaction-diffusion system. Using a mathematical  modeling approach we show that the system can produce a confined  expression of hyaluronan, but only if the inhibitory signal is  transferred to adjacent cells in exosomes. 

Recent experimental and theoretical studies suggest that crystallization and glass-like solidification are useful analogies for understanding cell ordering in confluent biological tissues. It remains unexplored how cellular ordering contributes to pattern formation during morphogenesis. With a computational model we show that a system of elongated, cohering biological cells can get dynamically arrested in a network pattern. Our model provides a new explanation for the formation of cellular networks in culture systems that exclude intercellular interaction via chemotaxis or mechanical traction.

Background Low-yield metabolism is a puzzling phenomenon in many unicellular and multicellular organisms. In abundance of glucose, many cells  use a highly wasteful fermentation pathway despite the availability of a high-yield pathway, producing many ATP molecules per glucose, e.g., oxidative phosophorylation. Some of these organisms, including the lactic acid bacterium Lactococcus lactis, downregulate their high-yield pathway in favor of the low-yield pathway. Other organisms, including Escherichia coli do not reduce the flux through the high-yield pathway,  employing the low-yield pathway in parallel with a fully active high-yield pathway. For what reasons do some species use the high-yield and low-yield pathway concurrently and what makes others downregulate the high-yield pathway? A classic rationale for metabolic fermentation is overflow metabolism. Because the throughput of metabolic pathways is limited, influx of glucose exceeding the pathway's throughput capacity is thought to be redirected into an alternative, low-yield pathway. This overflow metabolism rationale suggests that cells would only use fermentation once the high-yield pathway runs at maximum rate, but it cannot explain why cells would decrease the flux through the high-yield pathway.

Results Using flux balance analysis with molecular crowding (FBAwMC), a recent extension to flux balance analysis (FBA) that assumes that the total flux through the metabolic network is limited,  we investigate the differences between Saccharomyces cerevisiae and L. lactis that downregulate the high-yield pathway at increasing glucose concentrations, and E. coli, which keeps the high-yield pathway functioning at maximal rate. FBAwMC correctly predicts the metabolic switching mode in these three organisms, suggesting that metabolic network architecture is responsible for differences in metabolic switching mode. Based on our analysis, we expect gradual, 'overflow-like' switching behavior in organisms that have an additional energy-yielding pathway that does not consume NADH (e.g., acetate production in E. coli). Flux decrease through the high-yield pathway is expected in organisms in which the high-yield and low-yield pathways compete for NADH. In support of this analysis, a simplified model of metabolic switching suggests that the extra energy generated during acetate production produces an additional optimal growth mode that smoothens the metabolic switch in E. coli.

Conclusions Maintaining redox balance is key to explaining why some microbes decrease the flux through the high-yield pathway, while other microbes use 'overflow-like' low-yield metabolism. 

In the 1950s, embryology was conceptualized as four relatively independent problems: cell differentiation, growth, pattern formation and morphogenesis. The mechanisms underlying the first three traditionally have been viewed as being chemical in nature, whereas those underlying morphogenesis have usually been discussed in terms of mechanics. Often, morphogenesis and its mechanical processes have been regarded as subordinate to chemical ones. However, a growing body of evidence indicates that the biomechanics of cells and tissues affect in striking ways those phenomena often thought of as mainly under the control of cell-cell signalling. This accumulation of data has led to a revival of the mechano-transduction concept in particular, and of complexity in general, causing us now to consider whether we should retain the traditional conceptualization of development.The researchers’ semantic preferences for the terms ‘patterning’, ‘pattern formation’ or ‘morphogenesis’ can be used to describe three main ‘schools of thought’ which emerged in the late 1970s. In the ‘molecular school’, the term patterning is deeply tied to the positional information concept. In the ‘chemical school’, the term ‘pattern formation’ regularly implies reaction-diffusion models. In the ‘mechanical school’, the term ‘morphogenesis’ is more frequently used in relation to mechanical instabilities. Major differences among these three schools pertain to the concept of self-organization, and models can be classified as morphostatic or morphodynamic. Various examples illustrate the distorted picture that arises from the distinction among differentiation, growth, pattern formation and morphogenesis, based on the idea that the underlying mechanisms are respectively chemical or mechanical. Emerging quantitative approaches integrate the concepts and methods of complex sciences and emphasize the interplay between hierarchical levels of organization via mechano-chemical interactions. They draw upon recent improvements in mathematical and numerical morphogenetic models and upon considerable progress in collecting new quantitative data. This review highlights a variety of such models, which exhibit important advances, such as hybrid, stochastic and multiscale simulations.

Spatial organ arrangement plays an important role in flower development. The position and the identity of floral organs is influenced by various processes, in particular the expression of MADS-box transcription factors for identity and dynamics of the plant hormone auxin for positioning. We are currently integrating patterning processes of MADS and auxin into our computational models, based on interactions that are known from experiments, in order to get insight in how these define the floral body plan. The resulting computational model will help to explore hypothetical interactions between the MADS and auxin regulation networks in floral organ patterning. 

Combined with in vitro and in vivo experiments, mathematical and computational modeling are key to unraveling how mechanical and chemical signaling by endothelial cells coordinates their organization into capillary-like tubes. While in vitro and in vivo experiments can unveil the effects of for example environmental changes or gene knockouts, computational models provide a way to formalize and understand the mechanisms underlying these observations. This chapter reviews recent computational approaches to model angiogenesis, and discusses the insights they provide in the mechanisms of angiogenesis.

We introduce a new cell-based computational model of an in vitro assay of angiogenic sprouting from endothelial monolayers in fibrin matrices. Endothelial cells are modeled by the Cellular Potts Model, combined with continuum descriptions to model haptotaxis and proteolysis of the extracellular matrix. The computational model demonstrates how a variety of cellular structural properties and behaviors determine the dynamics of tube formation. We aim to extend this model to a multi-scale model in the sense that cells, extracellular matrix and cell-regulation are described at different levels of detail and feedback on each other. Finally we discuss how computational modeling, combined with in vitro and in vivo modeling steers experiments, and how it generates new experimental hypotheses and insights on the mechanics of angiogenesis.

Cell-based computational modeling and simulation are becoming invaluable tools in analyzing plant development. In a cell-based simulation model, the inputs are behaviors and dynamics of individual cells and the rules describing responses to signals from adjacent cells. The outputs are the growing tissues, shapes and cell-differentiation patterns that emerge from the local, chemical and biomechanical cell-cell interactions. Here, we present a step-by-step, practical tutorial for building cell-based simulations of plant development. We show how to build a model of a growing tissue, a reaction-diffusion system on a growing domain and an auxin transport model. The aim of VirtualLeaf is to make computational modeling better accessible to experimental plant biologists with relatively little computational background.

The study of transgenic Arabidopsis lines with altered vascular patterns has revealed key players in the venation process, but details of the vascularization process are still unclear, partly because most lines have only been assessed qualitatively. Therefore, quantitative analyses are required to identify subtle perturbations in the pattern and to test dynamic modeling hypotheses using biological measurements. We developed an online framework, designated Leaf Image Analysis Interface (LIMANI), in which venation patterns are automatically segmented and measured on dark-field images. Image segmentation may be manually corrected through use of an interactive interface, allowing supervision and rectification steps in the automated image analysis pipeline and ensuring high-fidelity analysis. This online approach is advantageous for the user in terms of installation, software updates, computer load and data storage. The framework was used to study vascular differentiation during leaf development and to analyze the venation pattern in transgenic lines with contrasting cellular and leaf size traits. The results show the evolution of vascular traits during leaf development, suggest a self-organizing mechanism for leaf venation patterning, and reveal a tight balance between the number of end-points and branching points within the leaf vascular network that does not depend on the leaf developmental stage and cellular content, but on the leaf position on the rosette. These findings indicate that development of LIMANI improves understanding of the interaction between vascular patterning and leaf growth. 

An intriguing phenomenon in plant development is the timing and positioning of lateral organ initiation, which is a fundamental aspect of plant architecture. Although important progress has been made in elucidating the role of auxin transport in the vegetative shoot to explain the phyllotaxis of leaf formation in a spiral fashion, a model study of the role of auxin transport in whorled organ patterning in the expanding floral meristem is not available yet. We present an initial simulation approach to study the mechanisms that are expected to play an important role. Starting point is a confocal imaging study of Arabidopsis floral meristems at consecutive time points during flower development. These images reveal auxin accumulation patterns at the positions of the organs, which strongly suggests that the role of auxin in the floral meristem is similar to the role it plays in the shoot apical meristem. This is the basis for a simulation study of auxin transport through a growing floral meristem, which may answer the question whether auxin transport can in itself be responsible for the typical whorled floral pattern. We combined a cellular growth model for the meristem with a polar auxin transport model. The model predicts that sepals are initiated by auxin maxima arising early during meristem outgrowth. These form a pre-pattern relative to which a series of smaller auxin maxima are positioned, which partially overlap with the anlagen of petals, stamens, and carpels. We adjusted the model parameters corresponding to properties of floral mutants and found that the model predictions agree with the observed mutant patterns. The predicted timing of the primordia outgrowth and the timing and positioning of the sepal primordia show remarkable similarities with a developing flower in nature.

In this contribution, I will explore the use of graph theory in analyzing the cellular configurations coming from our simulations of blood vessel growth. As a first step, we transform the simulated biological tissue into a graph that represents the adjacency relations of cells, focusing on topology. After outlining the cell-based algorithms we use to simulate blood vessel growth, we discuss preliminary explorations in the use of graph theoretic measures for characterizing blood vessel sprouting. We end by discussing some future developments in the discrete mathematics of biological morphogenesis.

Modellers are producing more and more complex models. Unless these models are sufficiently characterised and made available to the research community their reuse will be minimal, and reproducing simulation experiments incorporating them will prove problematic. Consensus on the content and form of experiment recipes that combine models and simulations will encourage model sharing and facilitate reuse.

A set of guidelines specifying the ‘Minimum Information About a Simulation Experiment' (MIASE) proposes a common set of information necessary to reproduce simulation experiments that incorporate quantitative models.

We have instantiated these guidelines in a web-based content management system. With our system you can create Simulation and Experiment Descriptions, enrich them with experimental data and annotate them with domain meta-information to facilitate classification, searching and cross referencing – all with the goal of reusing your models and reproducing your experimental results.

Systems Biology requires increasingly complex simulation  models. Effectively interpreting and building upon previous  simulation results is both difficult and time consuming. Thus,  simulation results often cannot be reproduced exactly; making it  difficult for other modellers to validate results and take the  next step in a simulation study.

The Simulation Experiment Description Mark-up Language (SEDML), a subset of the Minimum Information About a Simulation Experiment (MIASE) guidelines, promises to solve this problem by prescribing the form and content of the information required to reproduce simulation experiments. SEDML is detailed enough to enable automatic rerunning of simulation experiments.

Here, we present a web-based simulation-experiment repository lets modellers develop SEDML compliant simulation-experiment descriptions The system encourages modellers to annotate their experiments with text and images, experimental data and domain meta-information. These informal annotations aid organisation and classification of the simulations and provide rich search criteria. They complement SEDML's formal precision to produce simulation-experiment descriptions that can be understood by both men and machines. The system combines both human-readable and formal machine-readable content, thus ensuring exact reproducibility of the simulation results of a modelling study.

Lignin is a heteropolymer, which is thought to form in the cell wall by combinatorial radical coupling of monolignols. Here we present a simulation model of in vitro lignin polymerization, based on the combinatorial coupling theory, which allows us to predict the reaction conditions controlling the primary structure of lignin polymers. Our model predicts two controlling factors for the β-O-4-content of syringyl-guaiacyl lignins: the supply rate of monolignols and the relative amount of supplied sinapyl alcohol monomers. We have analyzed the in silico degradability of the resulting lignin polymers by cutting the resulting lignin polymers at β-O-4-bonds. These are cleaved in analytical methods used to study lignin composition, namely thioacidolysis and derivatization followed by reductive cleavage (DFRC), under pulping conditions, and in some lignocellulosic biomass pretreatments.

SHORT-ROOT (SHR) and SCARECROW (SCR) are required for stem cell maintenance in the Arabidopsis thaliana root meristem, ensuring its indeterminate growth. Mutation of SHR and SCR genes results in disorganization of the quiescent center and loss of stem cell activity, resulting in the cessation of root growth. This manuscript reports on the role of SHR and SCR in the development of leaves, which, in contrast to the root, have a determinate growth pattern and lack a persistent stem-cell niche. Our results demonstrate that inhibition of leaf growth in shr and scr mutants is not a secondary effect of the compromised root development, but is caused by an effect on cell division in the leaves: a reduced cell division rate and early exit of proliferation phase. Consistent with the observed cell division phenotype, the expression of SHR and SCR genes in leaves is closely associated with cell division activity in most cell types. The increased cell cycle duration is due to a prolonged S-phase duration, which is mediated by up-regulation of cell cycle inhibitors known to restrain the activity of the transcription factor, E2Fa. Therefore, we conclude that, in contrast to their specific role in cortex/endodermis differentiation and stem cell maintenance in the root, SHR and SCR primarily function as general regulators of cell proliferation in leaves.

Plant development is exceptionally flexible as manifested by its potential for organogenesis and regeneration, which are processes involving rearrangements of tissue polarities. Fundamental questions concern how individual cells can polarize in a coordinated manner to integrate into the multicellular context. In canalization models, the signaling molecule auxin acts as a polarizing cue, and feedback on the intercellular auxin flow is key for synchronized polarity rearrangements. We provide a novel mechanistic framework for canalization, based on up-to-date experimental data and minimal, biologically plausible assumptions. Our model combines the intracellular auxin signaling for expression of PINFORMED (PIN) auxin transporters and the theoretical postulation of extracellular auxin signaling for modulation of PIN subcellular dynamics. Computer simulations faithfully and robustly recapitulated the experimentally observed patterns of tissue polarity and asymmetric auxin distribution during formation and regeneration of vascular systems and during the competitive regulation of shoot branching by apical dominance. Additionally, our model generated new predictions that could be experimentally validated, highlighting a mechanistically conceivable explanation for the PIN polarization and canalization of the auxin flow in plants.

Plant organs, including leaves and roots, develop by means of a multi-level crosstalk between gene regulation, patterned cell division and cell expansion, and tissue mechanics. The multi-level regulatory mechanisms complicate classic molecular genetics or functional genomics approaches to biological development, because these methodologies implicitly assume a direct relation between genes and traits at the level of the whole plant or organ. Instead, understanding gene function requires insight into the role of gene products in regulatory networks, the conditions of gene expression, etc. This interplay is impossible to understand intuitively. Mathematical and computer modeling allows researchers to design new hypotheses and produce experimentally testable insights. However, the required mathematics and programming experience makes modeling poorly accessible to experimental biologists. Problem-solving environments provide biologically-intuitive in silico objects (cells, regulation networks) required for setting up a simulation, and present those to the user in terms of familiar, biological terminology. Here, we introduce the cell-based computer-modeling framework VirtualLeaf for plant tissue morphogenesis. The current version defines a set of biologically-intuitive C++ objects, including cells, cell walls, and diffusing and reacting chemicals, that provide useful abstractions for building biological simulations of developmental processes. We present a step-by-step introduction to building models with the VirtualLeaf, providing basic example models of leaf venation and meristem development. VirtualLeaf-based models provide a means for plant researchers to analyze the function of developmental genes in the context of the biophysics of growth and patterning. The VirtualLeaf is an ongoing Open Source software project that runs on Windows, Mac and Linux.

 

The different behaviors of colonies of two cell lines, ARO (thyroid carcinoma-derived cells) and MLP-29 (mouse liver progenitor cells), in response to hepatocyte growth factor (HGF) are described deducing suitable cellular Potts models (CPM). It is shown how increased motility and decreased adhesiveness are responsible for cell–cell dissociation and tissue invasion in the ARO cells. On the other hand, it is shown that, in addition to the biological mechanisms above, it is necessary to include directional persistence in cell motility and HGF diffusion to describe the scattering and the branching processes characteristic of MLP-29 cells. 

Cell-based, mathematical models help make sense of morphogenesis—i.e. cells organizing into shape and pattern—by capturing cell behavior in simple, purely descriptive models. Cell-based models then predict the tissue-level patterns the cells produce collectively. The first step in a cell-based modeling approach is to isolate sub-processes, e.g. the patterning capabilities of one or a few cell types in cell cultures. Cell-based models can then identify the mechanisms responsible for patterning in vitro. This review discusses two cell culture models of morphogenesis that have been studied using this combined experimental-mathematical approach: chondrogenesis (cartilage patterning) and vasculogenesis (de novo blood vessel growth). In both these systems, radically different models can equally plausibly explain the in vitro patterns. Quantitative descriptions of cell behavior would help choose between alternative models. We will briefly review the experimental methodology (microfluidics technology and traction force microscopy) used to measure responses of individual cells to their micro-environment, including chemical gradients, physical forces and neighboring cells. We conclude by discussing how to include quantitative cell descriptions into a cell-based model: the Cellular Potts model.

Blood vessels form either when dispersed endothelial cells (the cells lining the inner walls of fully formed blood vessels) organize into a vessel network (vasculogenesis), or by sprouting or splitting of existing blood vessels (angiogenesis). Although they are closely related biologically, no current model explains both phenomena with a single biophysical mechanism. Most computational models describe sprouting at the level of the blood vessel, ignoring how cell behavior drives branch splitting during sprouting. We present a cell-based, Glazier–Graner–Hogeweg model (also called Cellular Potts Model) simulation of the initial patterning before the vascular cords form lumens, based on plausible behaviors of endothelial cells. The endothelial cells secrete a chemoattractant, which attracts other endothelial cells. As in the classic Keller–Segel model, chemotaxis by itself causes cells to aggregate into isolated clusters. However, including experimentally observed VE-cadherin–mediated contact inhibition of chemotaxis in the simulation causes randomly distributed cells to organize into networks and cell aggregates to sprout, reproducing aspects of both de novo and sprouting blood-vessel growth. We discuss two branching instabilities responsible for our results. Cells at the surfaces of cell clusters attempting to migrate to the centers of the clusters produce a buckling instability. In a model variant that eliminates the surface–normal force, a dissipative mechanism drives sprouting, with the secreted chemical acting both as a chemoattractant and as an inhibitor of pseudopod extension. Both mechanisms would also apply if force transmission through the extracellular matrix rather than chemical signaling mediated cell–cell interactions. The branching instabilities responsible for our results, which result from contact inhibition of chemotaxis, are both generic developmental mechanisms and interesting examples of unusual patterning instabilities.

In this chapter we describe how the the Cellular Potts Model has been applied to problems in the biomedical field. Examples are given in epi- dermal biology, cancer and vasculogenesis. They demonstrate the strength of the CPM and its rich set of extensions, in elucidating biomedically important phenomena.

In 1969 Tsvi Sachs published his seminal hypothesis of vascular development in plants: the canalization hypothesis. A positive feedback loop between the flux of the phytohormone auxin and the cells' auxin transport capacity would canalize auxin progressively into discrete channels that then differentiate into vascular tissue. Recent experimental studies confirm the central role of polar auxin flux in plant vasculogenesis, but it is unclear if and by which mechanism plant cells could respond to auxin flux. In this Opinion article, we review auxin perception mechanisms and argue that these respond more likely to auxin concentrations than to auxin flux. We propose an alternative mechanism for polar auxin channeling, which is more consistent with recent molecular observations.

To gain performance, developers often build scientific applications in procedural languages, such as C or Fortran, which unfortunately reduces flexibility. To address this imbalance, the authors present CompuCell3D, a multitiered, flexible, and scalable problem-solving environment for morphogenesis simulations that's written in C++ using object-oriented design patterns. 

One of the reasons for the enormous success of the Glazier–Graner–Hogeweg Model (GGH ) model is that it is a framework for model building rather than a specific biological model. Thus new ideas constantly emerge for ways to extend it to describe new biological (and non-biological) phenomena. The GGH model automatically integrates exten-sions with the whole body of prior GGH work, a flexibility which makes it unusually simple and rewarding to work with. In this chapter we discuss some possible future directions to extend GGH modeling. We discuss off-lattice extensions to the GGH model, which can treat fluids and solids, new classes of model objects, approaches to increasing computational efficiency, parallelization, and new model-development platforms that will accelerate our ability to generate successful models. We also discuss a non-GGH, but GGH- inspired, model of plant development by Merks and collaborators, which uses the Hamiltonian and Monte-Carlo approaches of the GGH but solves them using Finite Element (FE) methods.

Studies of cell-matrix, cell-cell interaction, or angiogenesis on different matrices require simultaneous comparison of read-out parameters from differently treated companion cells. The culture conditions (cell number, temperature, volume of culture medium) in different chambers are not completely equalized using conventional methods. Herein we describe an innovative chamber which could resolve this problem by significantly improving the standardization of experimental conditions. The chamber was manufactured from a standard cell culture well by its division with a septum into two sections. We utilized the chamber and recently developed topological analysis to examine the effects of glycated matrices on the capillary network by endothelial cells. Glycated Collagen I resulted in dose-dependent changes to all measured topological characteristics of the capillary-like network, such as the number of branching points, number of meshes and total capillary length. These differences were observed only in neighbored compartments coated with different matrices, but not in the compartments coated with the same matrix. The novel chamber brings an opportunity for better standardization of experimental conditions and simultaneous observation of different experimental groups, reducing the possible effect of any systematic error.

Simulation is an important tool for understanding developmental mechanisms, but the technology is often too complex for biologists lacking higher computational skills. Using a tiered architecture, the developers of these problem-solving environment (PSE) prototypes ensure accessibility for users at all skill levels.

Vasculogenesis, the de novo growth of the primary vascular network from initially dispersed endothelial cells, is the first step in the development of the circulatory system in vertebrates. In the first stages of vasculogenesis, endothelial cells elongate and form a network-like structure, called the primary capillary plexus, which subsequently remodels, with the size of the vacancies between ribbons of endothelial cells coarsening over time. To isolate such intrinsic morphogenetic ability of endothelial cells from its regulation by long-range guidance cues and additional cell types, we use an in vitro model of human umbilical vein endothelial cells (HUVEC) in Matrigel. This quasi-two-dimensional endothelial cell culture model would most closely correspond to vasculogenesis in flat areas of the embryo like the yolk sac. Several studies have used continuum mathematical models to explore in vitro vasculogenesis: such models describe cell ensembles but ignore the endothelial cells' shapes and active surface fluctuations. While these models initially reproduce vascular-like morphologies, they eventually stabilize into a disconnected pattern of vascular "islands." Also, they fail to reproduce temporally correct network coarsening. Using a cell-centered computational model, we show that the endothelial cells' elongated shape is key to correct spatiotemporal in silico replication of stable vascular network growth. We validate our simulation results against HUVEC cultures using time-resolved image analysis and find that our simulations quantitatively reproduce in vitro vasculogenesis and subsequent in vitro remodeling. 

The formation of a polygonal configuration of proto-blood-vessels from initially dispersed cells, is the first step in the development of the circulatory system in vertebrates. This initial vascular network later expands to form new blood vessels, primarily via a sprouting mechanism. We review a range of recent results obtained with a Monte Carlo model of chemotactically migrating cells which can explain both de novo blood vessel growth and aspects of blood vessel sprouting. We propose that the initial network forms via a percolation-like instability depending on cell shape, or through an alternative contact-inhibition of motility mechanism which also reproduces aspects of sprouting blood vessel growth. 

Geen meter onder water ben ik geweest tijdens mijn onderzoek naar steenkoralen. Driedimensionaal groeiden ze in tientallen aan elkaar gekoppelde computers in de polders van de Watergraafsmeer, thuisbasis van de sectie Computational Science van de Universiteit van Amsterdam. Wachten op echte, langzaam groeiende koralen had mijn promotie laten duren tot ver na mijn pensioen. En waarom ook wachten? Een computermodel heeft grote voordelen, omdat je eenvoudig kan bepalen welke factoren bijdragen aan de groei.

Understanding external deciding factors in growth and morphology of reef corals is essential to elucidate the role of corals in marine ecosystems, and to explain their susceptibility to pollution and global climate change. Here, we extend on a previously presented model for simulating the growth and form of a branching coral and we compare the simulated morphologies to three-dimensional (3D) images of the coral species Madracis mirabilis. Simulation experiments and isotope analyses of M. mirabilis skeletons indicate that external gradients of dissolved inorganic carbon (DIC) determine the morphogenesis of branching, phototrophic corals. In the simulations we use a first principle model of accretive growth based on local interactions between the polyps. The only species-specific information in the model is the average size of a polyp. From flow tank and simulation studies it is known that a relatively large stagnant and diffusion dominated region develops within a branching colony. We have used this information by assuming in our model that growth is entirely driven by a diffusion-limited process, where DIC supply represents the limiting factor. With such model constraints it is possible to generate morphologies that are virtually indistinguishable from the 3D images of the actual colonies.

Explaining embryonic development of multicellular organisms requires insight into complex interactions between genetic regulation and physical, generic mechanisms at multiple scales. As more physicists move into developmental biology, we need to be aware of the cultural differences between the two fields, whose concepts of explanations and models traditionally differ, biologists aiming to identify the genetic pathways and expression patterns, physicists tending to look for generic underlying principles. Here we discuss how we can combine such biological and physical approaches into a cell-centered approach to developmental biology. Genetic information can only indirectly influence the morphology and physiology of multicellular organisms. DNA translates into proteins and regulatory RNA sequences, which steer the biophysical properties of cells, their response to signals from neighboring cells, and the production and properties of extracellular matrix (ECM). We argue that in many aspects of biological development, cells inner workings are irrelevant: what matters are the cell's biophysical properties, the signals it emits and its responses to extracellular signals. Thus we can separate questions about genetic regulation from questions of development. First, we ask what effects a gene network has on cell phenomenology, and how it operates. We then ask through which mechanisms such single-cell phenomenology directs multicellular morphogenesis and physiology. This approach treats the cell as the fundamental module of development. We discuss how this cell-centered approach which requires significant input from computational biophysics can assist and supplement experimental research in developmental biology. We review cell-centered approaches, focusing in particular on the Cellular Potts Model (CPM), and present the Tissue Simulation Toolkit, which implements the CPM.

Endothelium-derived microparticles emerged recently as a new marker of endothelial cell dysfunction. Increased levels of circulating microparticles have been documented in inflammatory disorders, diabetes mellitus, and many cardiovascular diseases. Perturbations of angiogenesis play an important role in the pathogenesis of these disorders. We have demonstrated previously that isolated endothelial microparticles (EMP) impair endothelial function in vitro, diminishing acetylcholine-induced vasorelaxation and nitric oxide production by rat aortic rings with a simultaneous increase in superoxide production. Herein, using the Matrigel assay of angiogenesis in vitro and a topological analysis of the capillary-like network by Human Umbilical Vein Endothelial Cells (HUVEC), we investigated the effects of EMP on the formation of the vascular network. All parameters of angiogenesis were affected by treatment for 48 hours with isolated EMP in concentrations 105/ml, but not at 103/ml or 104/ml. The effects included: decreased total capillary length (24%), decreased number of meshes (45%), decreased branching points (36%), and increased mesh area (38%). The positional and topological order indicated that EMP affect angiogenic parameters uniformly over the capillary network. Treatment with the cell-permeable SOD-mimetic MnTBAP partially or completely restored all parameters of angiogenesis affected by EMP. EMP reduced the cell proliferation rate and increased the apoptosis rate in a time- and dose dependent manner, a phenomenon which was also prevented by MnTBAP. Our data demonstrate that endothelial microparticles have considerable impact on angiogenesis in vitro and may be an important contributor to the pathogenesis of diseases that are accompanied by impaired angiogenesis.  

The morphogenesis of colonial stony corals is the result of the collective behaviour of many coral polyps depositing coral skeleton on top of the old skeleton on which they live. Yet, models of coral growth often consider the polyps as a single continuous surface. In the present work, the polyps are modelled individually. Each polyp takes up resources, deposits skeleton, buds off new polyps and dies. In this polyp oriented model, spontaneous branching occurs. We argue that branching is caused by a so called "polyp fanning effect" by which polyps on a convex surface have a competitive advantage relative to polyps on a flat or concave surface. The fanning effect generates a more potent branching mechanism than the Laplacian growth mechanism that we have studied previously (J. Theor. Biol. 224 (2003) 153). We discuss the application of the polyp oriented model to the study of environmentally driven morphological plasticity in stony corals. In a few examples we show how the properties of the individual polyps influence the whole colony morphology. In our model, the spacing of polyps influences the thickness of coral branches and the overall compactness of the colony. Density variations in the coral skeleton may also be important for the whole colony morphology, which we address by studying two variants of the model. Finally, we discuss the importance of small scale resource translocation in the coral colony and its effects on the morphology of the colony.

We introduce a Cellular Potts model (a cellular-automaton-based Monte-Carlo model) of in vitro capillary development, or angiogenesis. Our model derives from a recent continuum model, which assumes that vascular endothelial cells chemotactically attract each other. Our discrete model is ``cell based.'' Modeling the cells individually allows us to assign different physicochemical properties to each cell and to study how these properties affect the vascular pattern. Using the model, we assess the roles of intercellular adhesion, cell shape and chemoattractant saturation in  in vitro capillary development. We discuss how our computational model can serve as a tool for experimental biologists to “pre-test” hypotheses and to suggest new experiments.

In the diffusion-limited aggregation (DLA) model, pioneered by Witten and Sander (Phys. Rev. Lett. 47, 1400 (1981)), diffusing particles irreversibly attach to a growing cluster which is initiated with a single solid seed. This process generates clusters with a branched morphology. Advection-diffusion-limited aggregation (ADLA) is a straightforward extension to this model, where the transport of the aggregating particles not only depends on diffusion, but also on a fluid flow. The authors studying two-dimensional and three-dimensional ADLA in laminar flows reported that clusters grow preferentially against the flow direction. The internal structure of the clusters was mostly reported to remain unaffected, except by Kaandorp et al. (Phys. Rev. Lett. 77, 2328 (1996)) who found compact clusters ``as the flow becomes more important''. In the present paper we present three-dimensional simulations of ADLA. We did not find significant effects of low Reynolds-number advection on the cluster structure. The contradicting results by Kaandorp et al. (1996) were recovered only when the relaxation into equilibrium of the advection-diffusion field was too slow, in combination with the synchronous addition of multiple particles. 

In stony corals it is often observed that specimens collected from a sheltered growth site have more open and more thinly branched growth forms than specimens of the same species from more exposed growth sites, where stronger water currents are found. This observation was explained using an abiotic computational model inspired by coral growth, in which the growth velocity depended locally on the absorption of a resource dispersed by advection and diffusion (Kaandorp & Sloot (2001), J. Theor. Biol 209, 257-274). In that model a morphological range was found; as the Péclet-number (indicating the relative importance of advective and diffusive nutrient transport) was increased, more compact and spherical growth forms were found. Two unsatisfactory items have remained in this model, which we address in the present paper. First, an explicit curvature rule was responsible for branching. In this work we show that the curvature rule is not needed: the model exhibits spontaneous branching, provided that the resource field is computed with enough precision. Second, previously no explanation was given for the morphological range found in the simulations. Here we show that such an explanation is given by the conditions under which spontaneous branching occurs in our model, in which the compactness of the growth forms depends on the ratio of the rates of growth and nutrient transport. We did not find an effect of flow. This suggests that the computational evidence that hydrodynamics influences the compactness of corals in laminar flows may not be conclusive. We discuss the applicability of the Laplacian growth paradigm to understand coral growth is discussed.

Apart from experimental and theoretical approaches, computer simulation is an important tool in testing hypotheses about stony coral growth. However, the construction and use of such simulation tools needs extensive computational skills and knowledge that is not available to most research biologists. Problems solvings environments (PSEs) aim to provide a framework that hides implementation details and allows the user to formulate and analyse a problem in the language of the subject area. We have developed a prototypical PSE to study the morphogenesis of corals using a multi-model approach. In this paper we describe the design and implementation of this PSE, in which simulations of the coral's shape and its environment have been combined. We will discuss the relevance of our results for the future development of PSEs for studying biological growth and morphogenesis.

We numerically validate the moment propagation method for advection-diffusion in a Lattice Boltzmann simulation against the analytic Taylor-Aris prediction for dispersion in a three-dimensional Poiseuille flow. Good agreement between simulation and the theory is found, with relative errors smaller than 2%.

The Péclet number limits on the moment propagation method are studied, and maximum parameter values are obtained. We show that a modification of the moment-propagation method allows advection-diffusion simulations with higher Péclet numbers, in particular in the low Reynolds number limit.

A three-dimensional model of diffusion limited coral growth is introduced. As opposed to previous models, in this model we take a “polyp oriented” approach. Here, coral morphogenesis is the result of the collective behaviour of the individual coral polyps. In the polyp oriented model, branching occurs spontaneously, as opposed to previous models in which an explicit rule was responsible for branching. We discuss the mechanism of branching in our model. Also, the effects of polyp spacing on the coral morphology are studied.